Sobre os números P-ádicos: aspectos históricos, matemáticos e epistemológicos
DOI:
10.47976/RBHM2024v24n481-32Palavras-chave:
Números p-ádicos, História da Matemática, Intuição, Topologia dos números p-ádicosResumo
Reconhecidamente, o processo de engendramento evolutivo matemático que concorreu para a evolução e a determinação de bases sólidas para a noção de número real constitui um emblemático divisor de águas para a Matemática no século XIX. Assim, a partir de um conhecimento atual de vários métodos e formas de determinação e do completamento do corpo dos números racionais, a despeito do método empregado, podemos indicar a natureza de um corpo arquimediano e completo que nominamos por conjunto dos números reais. Não obstante, o presente trabalho apresenta uma discussão de elementos históricos, matemáticos e epistemológicos sobre uma outra classe de números que representa, também, um completamento do conjunto dos números racionais e que se denota por , onde é um número primo. Dessa forma, os números p-ádicos são apresentados, tendo em vista proporcionar ao leitor uma vital compreensão do seu papel epistemológico que concorre para um entendimemo diferenciado e substancialmente ampliado para a noção abstrata e basilar em Matemática do que conhecemos ordinariamente por número.
Downloads
Não há dados estatísticos.
Métricas
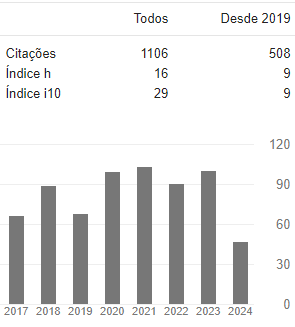
Carregando Métricas ...
Referências
ALVES, Francisco, R. V. (2017). Fórmula de de moivre, ou de binet ou de lamé: demonstrações e generalidades sobre a sequência generalizada de fibonacci – SGF. Revista Brasileira de História da Matemática, v, 17, nº 33, 1 – 16.
AMICE, Ivete. (1975). Le nombres p-adique. Paris: Presses Universitaire. Disponível em: https://www-fourier.ujf-grenoble.fr/~panchish/GDL16/amice_les-nombres-p-adiques%20%5B2755599%5D.pdf
AÇIKGÖZ, Mehmet; ASLAN,Nurgül; Köşkeroğlu, Nurten e Aracı, Serkan. (2009). p-Adic Approach to Linear 2-Normed Spaces. Mathematica Moravica, v. 13, nº 2, 7 – 21. Disponível em: http://scindeks-clanci.ceon.rs/data/pdf/1450-5932/2009/1450-59320902007A.pdf
ADERBURG, Drew. (2002). A Mathematical Seduction. Math Horizons, 9:3, 12-15. Disponível em: https://web.williams.edu/Mathematics/eburger/BurgerMathHorizons.pdf
BELAIR, Luc. (2012). Panorama of p-adic model theory, Annales des Sciences Mathematiques du Quebec, nº 36, p. 43-75, 2012. Disponível em: http://www.logique.jussieu.fr/modnet/Publications/Introductory%20Notes%20and%20surveys/Belair.pdf
BOREVICH, Z. I.; SHAFAREVICH, I. R. (1966). Number Theory. New York: Academic Press.
BURGER, Edward, B. (2000). Exploring the number jungle: a journey into diophantine analysis. New York: Americal Mathematical Society.
CĂLIN, Mureşan Alexe. (2006). Non-Archimedian Fields. Topological Properties of Zp, Qp (p-adics Numbers). Matematică - Informatică – Fizică, v. 63, nº 2, 43 – 48. Disponível em: http://bmif.unde.ro/docs/20062/7%20MuresanA.pdf
CARVALHO, Maria Pires de; LOURENÇO, João Nuno P. (2015). Convergência de séries p-ádicas. Boletim da Sociedade Portuguesa de Matemática, 1 – 30, Disponível em: https://repositorio-aberto.up.pt/bitstream/10216/90725/2/104694.pdf
CHINEA, C. (2000). Matemática. ¿qué son los números p-adicos?. carlos s. chinea, octubr. Divulgación de la matemática en la red. 1 – 5. Disponível em: https://casanchi.com/casanchi_2000/19_padicos01.pdf
CORNELISSEN, Gunther; KATO, Fumiharu. (2005). The p-adic icosaedron. Notes in American Mathematical Association - AMS. v. 52, nº 7, 720 – 727. Disponível em: https://www.ams.org/notices/200507/fea-cornelissen.pdf
COHEN, Henri. (2007). Number Theory Volume I: Tools and Diophantine Equations. New York: Springer.
CORRY, Leo. (2004). Modern Algebra and the Rise of Mathematical Structures, Basel and Boston, Birkhäuser.
CUOCO, Albert. A. Visualizing the p-adic integers. American Mathematical Monthly. v. 98, nº 4, 355 – 364. Disponível em: https://www.jstor.org/stable/pdf/2323809.pdf?refreqid=excelsior%3Ada3401d345ac7e62ccac8f6378fb2cfc
CROMPTON, Catherine (2007) Some Geometry of the p-adic rationals. Rose-Hulman Undergraduate Mathematics Journal: v. 8 : Iss. 1 , Article 2. 1 – 13. Disponível em: https://scholar.rose-hulman.edu/cgi/viewcontent.cgi?referer=https://www.google.com.br/&httpsredir=1&article=1183&context=rhumj
DELAHAYE. Jean-Paul. (2001). Les nombres infinit vers la gauche. Pour la Science, nº 279, 100 – 104. Disponível em: http://www.lifl.fr/~jdelahay/pls/081.pdf
EHRLICH. Philip. (2006). The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Emergence of non-Archimedean Systems of Magnitudes. The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Archive History in Exact Sciences. V. 60, 1 – 121. Disponível em: http://prima.lnu.edu.ua/faculty/mechmat/Departments/MFAUKR/attachments/erlich.pdf
FERREIRA, Jamil. (2010). A construção dos números. Textos Universitários. Rio de Janeiro: SBM.
GOLDBLATT, Robert. (1998). Lectures on the Hyprreeals numbers: an introduction to non standard analisys. New York: Springer.
GOUVÊA, F. Q. (1997). p-adic numbers. New York: Springer.
GUSMÃO, Italo, B. (2016). Números p-ádicos. Dissertação de Mestrado Profissional PROFMAT, João Pessoa: UFPB.. Disponível em: https://repositorio.ufpb.br/jspui/bitstream/tede/9337/2/arquivototal.pdf
GAUTSCHI, Walter. Alessandro M. (2002). Ostrowski (1893–1986): la sua vita e le opere. Boll. Docenti Matem. v. 45, 9–19. Disponível em: https://www.cs.purdue.edu/homes/wxg/AMOital.pdf
HASSE, Helmut. (1980). Number Theory. Springer: New York.
HEFEZ, Abramo. (2013). Um curso de Àlgebra, v. 1, Rio de Janeiro: SBM.
HOLLY, Jan E. (2001). Pictures of Ultrametric Spaces, the p-adic Numbers, and Valued Fields. The mathematical association of America. October, 721 – 728. Disponível em: https://www.colby.edu/math/faculty/Faculty_files/hollydir/Holly01.pdf
HUAMAN, Ronald M. (2015). Raıces p-adicas de la unidad. (Tesis de Maestrıa). Pontificia Universidad del Perú: Lima. Disponível em: http://tesis.pucp.edu.pe/repositorio/bitstream/handle/123456789/6415/MAS_HUAMAN_RONALD_PADICAS.pdf;sequence=1
LAGES, Elon. (2010). Curso de Análise, v. 1. Rio de Janeiro: SBM.
LAPIDUS, Michel L.; HUNG. Lu. (2011). The Geometry of p-Adic Fractal Strings: A Comparative Survey. Contemporary Mathematics, v. 551, 163 – 206. Disponível em: http://www.math.ucr.edu/~lapidus/papers/ContMath/GeometrypAdicStringsSurvey10893.pdf
LAPIDUS, Michel L.; HUNG. Lu. (2008). Nonarchimedean Cantor set and string. Journal of Fixed Point Theory and Applications. v. 4, p. 1 – 10. Disponível em: https://pdfs.semanticscholar.org/6dee/45208234f48fe156d174d4ad56b17363d2ef.pdf
LEGUAY, Mathieu; HENRI, Joseph. (1992). Distance p-adique : une distance qui n'est pas habituelle. MATh.en.JEANS” au Palais de la Découverte. 33 – 36. Disponível em: http://mathenjeans.free.fr/amej/edition/actes/actespdf/92033036.pdf
MARCOS, José. E. (2006). The algebraic closure of a p-adic number field is a complete topological field. Mathematica Slovaca,v. 56, No. 3, p. 317—331. Disponível em: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.1010.7259&rep=rep1&type=pdf
MASIAS, Henry Zorrilla. (2011). Complecion no arquimedeana. Pro Mathematica, v. 25, 49-50. Disponível em: http://revistas.pucp.edu.pe/index.php/promathematica/article/viewFile/2666/2610
NARICI, Lawrence; BECKENSTEIN, Edward. (1981). Strange Terrain--Nonarchimedean Spaces. The American Mathematical Monthly, Vol. 88, No. 9 (Nov., 1981), pp. 667-676. Disponível em: https://www.jstor.org/stable/pdf/2320670.pdf
OSTROWSKI, A. (1916). Uber einige L¨osungen der Funktionalgleichung ¨ φ(x)φ(y) = φ(xy)”, Acta Mathematica (2nd ed.), nº 41, no. 1, 271–284. Disponível em: https://projecteuclid.org/euclid.acta/1485887472
KATO, Kazuya; KUROKAWA, Nobushige; SAITO, Takeshi, (2000). Number theory 1: Fermat’s dream, Translations of Mathematical Monographs, vol. 186, American Mathematical Society, Providence, RI.
KATOK, S. (2007). p-adic analysis compared with real. Student mathematical library, American Mathematical Soc.
KOBLITZ, Neal. (1984). p-adic Numbers, p-adic Analysis, and Zeta- Functions. New York: Springer.
KHRENNIKOV, Andrei; KOTOVICHM, Nikolay. (2017). Image Segmentation with the Aid of the p-Adic Metrics. In: TONI, Bourama. New Trends and Advanced Methods in Interdisciplinary Mathematical Sciences. 143 – 155.
KUMAR, Ashish; RANI, Mamta; CHUGH, Renu. (2013). New 5-adic Cantor sets and fractal string. SpringerPlus, 1 – 7. Disponível em: https://link.springer.com/content/pdf/10.1186%2F2193-1801-2-654.pdf
LEWIS, Robert Y. (2019). A formal proof of Hensel’s lemma over the p-adic integers. CPP’19, January p. 14–15, Lisbon, PT. Disponível em: http://robertylewis.com/padics/padics.pdf
NATARAJAN, P N; RANGANATHAN, K. N. (2000). A Geometry in which all Triangles are Isosceles An Introduction to Non-Archimedean Analysis. Resonance, October, 32 – 42. Disponível em: https://www.ias.ac.in/article/fulltext/reso/005/10/0032-0042
NEUKIRCH, J. (1990). The p-Adic numbers. EBBINGHAUS, H.-D. Numbers. New York: Springer. 155 – 179.
OLIVEIRA, Graciano. (2009). O corpo dos p-ádicos. Gazeta Matemática, Dezembro, 7 – 18. Disponível em: http://gazeta.spm.pt/getArtigo?gid=258
ORBEGOSO, Jorge Luis Rojas. Completitud y clausura algebraica de campos P-ádicos. Universidad nacional mayor de san marcos. Perú: Lima, 2016. Disponível em: http://cybertesis.unmsm.edu.pe/bitstream/handle/cybertesis/6315/Rojas_oj.pdf?sequence=1
PITANEN, Matti. (2015). How Imagination Could Be Realized p-Adically?. Journal of Consciousness Exploration & Research, v 6, nº 6, 354 – 356. Disponível em: https://jcer.com/index.php/jcj/article/viewFile/468/488
REDDY, B. Surender; SHANKARAIAH, D. (2013). On i-cauchy sequences in p-adic linear 2-normed spaces. International Journal of Pure and Applied Mathematics. v. 89, nº 4, 483 – 496. Disponivel em: https://ijpam.eu/contents/2013-89-4/4/4.pdf
RIBENBOIM, Paulo. (1999). The Theory of Classical Valuations. New York: Springer.
ROBERT, Alain. (1996). Qu´est-qe que le nombres p- ádique? Societé des enseignants neuchâtelois des Sciences. Bulletin, setembre, 5 – 12. Disponível em: http://www.sens-neuchatel.ch/bulletin/anciens-no-pdf/BULL18.PDF
ROZIKOV, U. A. (2013). What are p-Adic Numbers? What are They Used for?. Asia Pacific Mathematics Newsletter. v. 3, nº 4, 1 – 6, October. Disponível em: http://www.asiapacific-mathnews.com/03/0304/0001_0006.pdf
SCHIKHOF W. H. (1984). Ultrametric Calculus: An introduction to p-adic Analysis. Cambridge: University Press.
SCHLICHENMAIER, Martin. (2007). An Introduction to Riemann Surfaces, Algebraic Curves and Moduli Spaces. Springer: New York.
SILVERMANM, Joseph H. (2013). What is the p-adic Mandelbrot Set?. Notices of the AMS, setember, v. 60, nº 8, 1048 – 1050. Disponível em: http://www.ams.org/notices/201308/201308-full-issue.pdf
STEUDING, Jorn. (2002). The world of p-adic numbers and p-adic functions. Faculty of Physics and Mathematics. nº 5, 90 – 107. Disponível em: http://siauliaims.su.lt/pdfai/2002/STEUD-02.pdf
TEITELBAUM. Jeremy. (1995). The Geometry of p-adic Symmetric Spaces. Notice in American Mathematical Monthly. v. 42, nº 10, 1120 – 1126. Disponível em: https://www.ams.org/notices/199510/teitelbaum.pdf
TELLER, Jacek. (2012). Newton polygons on p-adic number fields. (dissertation in Arts and Mathematics). East Caroline University. Disponível em: http://thescholarship.ecu.edu/bitstream/handle/10342/3848/Teller_ecu_0600M_10676.pdf?sequence=1
TORRES, Sergio Carrillo; AMAYA, Carlos Hurtado. (2001). Una introduccion a los numeros p-adicos. Memorias XVIII encuentro de geometrıa y VI de aritmetica, 359 – 369. Disponível em: http://funes.uniandes.edu.co/5615/1/CarrilloUnaintroduccionGeometr%C3%ADa2008.PDF
VACCON. Tristan. (2015). Précision p-adique. (thésis de doctorat). Rennes: Université de Rennes. Disponível em: https://tel.archives-ouvertes.fr/tel-01205269v2/document
ALVES, Francisco, R. V. (2017). Fórmula de de moivre, ou de binet ou de lamé: demonstrações e generalidades sobre a sequência generalizada de fibonacci – SGF. Revista Brasileira de História da Matemática, v, 17, nº 33, 1 – 16.
AMICE, Ivete. (1975). Le nombres p-adique. Paris: Presses Universitaire. Disponível em: https://www-fourier.ujf-grenoble.fr/~panchish/GDL16/amice_les-nombres-p-adiques%20%5B2755599%5D.pdf
AÇIKGÖZ, Mehmet; ASLAN,Nurgül; Köşkeroğlu, Nurten e Aracı, Serkan. (2009). p-Adic Approach to Linear 2-Normed Spaces. Mathematica Moravica, v. 13, nº 2, 7 – 21. Disponível em: http://scindeks-clanci.ceon.rs/data/pdf/1450-5932/2009/1450-59320902007A.pdf
ADERBURG, Drew. (2002). A Mathematical Seduction. Math Horizons, 9:3, 12-15. Disponível em: https://web.williams.edu/Mathematics/eburger/BurgerMathHorizons.pdf
BELAIR, Luc. (2012). Panorama of p-adic model theory, Annales des Sciences Mathematiques du Quebec, nº 36, p. 43-75, 2012. Disponível em: http://www.logique.jussieu.fr/modnet/Publications/Introductory%20Notes%20and%20surveys/Belair.pdf
BOREVICH, Z. I.; SHAFAREVICH, I. R. (1966). Number Theory. New York: Academic Press.
BURGER, Edward, B. (2000). Exploring the number jungle: a journey into diophantine analysis. New York: Americal Mathematical Society.
CĂLIN, Mureşan Alexe. (2006). Non-Archimedian Fields. Topological Properties of Zp, Qp (p-adics Numbers). Matematică - Informatică – Fizică, v. 63, nº 2, 43 – 48. Disponível em: http://bmif.unde.ro/docs/20062/7%20MuresanA.pdf
CARVALHO, Maria Pires de; LOURENÇO, João Nuno P. (2015). Convergência de séries p-ádicas. Boletim da Sociedade Portuguesa de Matemática, 1 – 30, Disponível em: https://repositorio-aberto.up.pt/bitstream/10216/90725/2/104694.pdf
CHINEA, C. (2000). Matemática. ¿qué son los números p-adicos?. carlos s. chinea, octubr. Divulgación de la matemática en la red. 1 – 5. Disponível em: https://casanchi.com/casanchi_2000/19_padicos01.pdf
CORNELISSEN, Gunther; KATO, Fumiharu. (2005). The p-adic icosaedron. Notes in American Mathematical Association - AMS. v. 52, nº 7, 720 – 727. Disponível em: https://www.ams.org/notices/200507/fea-cornelissen.pdf
COHEN, Henri. (2007). Number Theory Volume I: Tools and Diophantine Equations. New York: Springer.
CORRY, Leo. (2004). Modern Algebra and the Rise of Mathematical Structures, Basel and Boston, Birkhäuser.
CUOCO, Albert. A. Visualizing the p-adic integers. American Mathematical Monthly. v. 98, nº 4, 355 – 364. Disponível em: https://www.jstor.org/stable/pdf/2323809.pdf?refreqid=excelsior%3Ada3401d345ac7e62ccac8f6378fb2cfc
CROMPTON, Catherine (2007) Some Geometry of the p-adic rationals. Rose-Hulman Undergraduate Mathematics Journal: v. 8 : Iss. 1 , Article 2. 1 – 13. Disponível em: https://scholar.rose-hulman.edu/cgi/viewcontent.cgi?referer=https://www.google.com.br/&httpsredir=1&article=1183&context=rhumj
DELAHAYE. Jean-Paul. (2001). Les nombres infinit vers la gauche. Pour la Science, nº 279, 100 – 104. Disponível em: http://www.lifl.fr/~jdelahay/pls/081.pdf
EHRLICH. Philip. (2006). The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Emergence of non-Archimedean Systems of Magnitudes. The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Archive History in Exact Sciences. V. 60, 1 – 121. Disponível em: http://prima.lnu.edu.ua/faculty/mechmat/Departments/MFAUKR/attachments/erlich.pdf
FERREIRA, Jamil. (2010). A construção dos números. Textos Universitários. Rio de Janeiro: SBM.
GOLDBLATT, Robert. (1998). Lectures on the Hyprreeals numbers: an introduction to non standard analisys. New York: Springer.
GOUVÊA, F. Q. (1997). p-adic numbers. New York: Springer.
GUSMÃO, Italo, B. (2016). Números p-ádicos. Dissertação de Mestrado Profissional PROFMAT, João Pessoa: UFPB.. Disponível em: https://repositorio.ufpb.br/jspui/bitstream/tede/9337/2/arquivototal.pdf
GAUTSCHI, Walter. Alessandro M. (2002). Ostrowski (1893–1986): la sua vita e le opere. Boll. Docenti Matem. v. 45, 9–19. Disponível em: https://www.cs.purdue.edu/homes/wxg/AMOital.pdf
HASSE, Helmut. (1980). Number Theory. Springer: New York.
HEFEZ, Abramo. (2013). Um curso de Àlgebra, v. 1, Rio de Janeiro: SBM.
HOLLY, Jan E. (2001). Pictures of Ultrametric Spaces, the p-adic Numbers, and Valued Fields. The mathematical association of America. October, 721 – 728. Disponível em: https://www.colby.edu/math/faculty/Faculty_files/hollydir/Holly01.pdf
HUAMAN, Ronald M. (2015). Raıces p-adicas de la unidad. (Tesis de Maestrıa). Pontificia Universidad del Perú: Lima. Disponível em: http://tesis.pucp.edu.pe/repositorio/bitstream/handle/123456789/6415/MAS_HUAMAN_RONALD_PADICAS.pdf;sequence=1
LAGES, Elon. (2010). Curso de Análise, v. 1. Rio de Janeiro: SBM.
LAPIDUS, Michel L.; HUNG. Lu. (2011). The Geometry of p-Adic Fractal Strings: A Comparative Survey. Contemporary Mathematics, v. 551, 163 – 206. Disponível em: http://www.math.ucr.edu/~lapidus/papers/ContMath/GeometrypAdicStringsSurvey10893.pdf
LAPIDUS, Michel L.; HUNG. Lu. (2008). Nonarchimedean Cantor set and string. Journal of Fixed Point Theory and Applications. v. 4, p. 1 – 10. Disponível em: https://pdfs.semanticscholar.org/6dee/45208234f48fe156d174d4ad56b17363d2ef.pdf
LEGUAY, Mathieu; HENRI, Joseph. (1992). Distance p-adique : une distance qui n'est pas habituelle. MATh.en.JEANS” au Palais de la Découverte. 33 – 36. Disponível em: http://mathenjeans.free.fr/amej/edition/actes/actespdf/92033036.pdf
MARCOS, José. E. (2006). The algebraic closure of a p-adic number field is a complete topological field. Mathematica Slovaca,v. 56, No. 3, p. 317—331. Disponível em: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.1010.7259&rep=rep1&type=pdf
MASIAS, Henry Zorrilla. (2011). Complecion no arquimedeana. Pro Mathematica, v. 25, 49-50. Disponível em: http://revistas.pucp.edu.pe/index.php/promathematica/article/viewFile/2666/2610
NARICI, Lawrence; BECKENSTEIN, Edward. (1981). Strange Terrain--Nonarchimedean Spaces. The American Mathematical Monthly, Vol. 88, No. 9 (Nov., 1981), pp. 667-676. Disponível em: https://www.jstor.org/stable/pdf/2320670.pdf
OSTROWSKI, A. (1916). Uber einige L¨osungen der Funktionalgleichung ¨ φ(x)φ(y) = φ(xy)”, Acta Mathematica (2nd ed.), nº 41, no. 1, 271–284. Disponível em: https://projecteuclid.org/euclid.acta/1485887472
KATO, Kazuya; KUROKAWA, Nobushige; SAITO, Takeshi, (2000). Number theory 1: Fermat’s dream, Translations of Mathematical Monographs, vol. 186, American Mathematical Society, Providence, RI.
KATOK, S. (2007). p-adic analysis compared with real. Student mathematical library, American Mathematical Soc.
KOBLITZ, Neal. (1984). p-adic Numbers, p-adic Analysis, and Zeta- Functions. New York: Springer.
KHRENNIKOV, Andrei; KOTOVICHM, Nikolay. (2017). Image Segmentation with the Aid of the p-Adic Metrics. In: TONI, Bourama. New Trends and Advanced Methods in Interdisciplinary Mathematical Sciences. 143 – 155.
KUMAR, Ashish; RANI, Mamta; CHUGH, Renu. (2013). New 5-adic Cantor sets and fractal string. SpringerPlus, 1 – 7. Disponível em: https://link.springer.com/content/pdf/10.1186%2F2193-1801-2-654.pdf
LEWIS, Robert Y. (2019). A formal proof of Hensel’s lemma over the p-adic integers. CPP’19, January p. 14–15, Lisbon, PT. Disponível em: http://robertylewis.com/padics/padics.pdf
NATARAJAN, P N; RANGANATHAN, K. N. (2000). A Geometry in which all Triangles are Isosceles An Introduction to Non-Archimedean Analysis. Resonance, October, 32 – 42. Disponível em: https://www.ias.ac.in/article/fulltext/reso/005/10/0032-0042
NEUKIRCH, J. (1990). The p-Adic numbers. EBBINGHAUS, H.-D. Numbers. New York: Springer. 155 – 179.
OLIVEIRA, Graciano. (2009). O corpo dos p-ádicos. Gazeta Matemática, Dezembro, 7 – 18. Disponível em: http://gazeta.spm.pt/getArtigo?gid=258
ORBEGOSO, Jorge Luis Rojas. Completitud y clausura algebraica de campos P-ádicos. Universidad nacional mayor de san marcos. Perú: Lima, 2016. Disponível em: http://cybertesis.unmsm.edu.pe/bitstream/handle/cybertesis/6315/Rojas_oj.pdf?sequence=1
PITANEN, Matti. (2015). How Imagination Could Be Realized p-Adically?. Journal of Consciousness Exploration & Research, v 6, nº 6, 354 – 356. Disponível em: https://jcer.com/index.php/jcj/article/viewFile/468/488
REDDY, B. Surender; SHANKARAIAH, D. (2013). On i-cauchy sequences in p-adic linear 2-normed spaces. International Journal of Pure and Applied Mathematics. v. 89, nº 4, 483 – 496. Disponivel em: https://ijpam.eu/contents/2013-89-4/4/4.pdf
RIBENBOIM, Paulo. (1999). The Theory of Classical Valuations. New York: Springer.
ROBERT, Alain. (1996). Qu´est-qe que le nombres p- ádique? Societé des enseignants neuchâtelois des Sciences. Bulletin, setembre, 5 – 12. Disponível em: http://www.sens-neuchatel.ch/bulletin/anciens-no-pdf/BULL18.PDF
ROZIKOV, U. A. (2013). What are p-Adic Numbers? What are They Used for?. Asia Pacific Mathematics Newsletter. v. 3, nº 4, 1 – 6, October. Disponível em: http://www.asiapacific-mathnews.com/03/0304/0001_0006.pdf
SCHIKHOF W. H. (1984). Ultrametric Calculus: An introduction to p-adic Analysis. Cambridge: University Press.
SCHLICHENMAIER, Martin. (2007). An Introduction to Riemann Surfaces, Algebraic Curves and Moduli Spaces. Springer: New York.
SILVERMANM, Joseph H. (2013). What is the p-adic Mandelbrot Set?. Notices of the AMS, setember, v. 60, nº 8, 1048 – 1050. Disponível em: http://www.ams.org/notices/201308/201308-full-issue.pdf
STEUDING, Jorn. (2002). The world of p-adic numbers and p-adic functions. Faculty of Physics and Mathematics. nº 5, 90 – 107. Disponível em: http://siauliaims.su.lt/pdfai/2002/STEUD-02.pdf
TEITELBAUM. Jeremy. (1995). The Geometry of p-adic Symmetric Spaces. Notice in American Mathematical Monthly. v. 42, nº 10, 1120 – 1126. Disponível em: https://www.ams.org/notices/199510/teitelbaum.pdf
TELLER, Jacek. (2012). Newton polygons on p-adic number fields. (dissertation in Arts and Mathematics). East Caroline University. Disponível em: http://thescholarship.ecu.edu/bitstream/handle/10342/3848/Teller_ecu_0600M_10676.pdf?sequence=1
TORRES, Sergio Carrillo; AMAYA, Carlos Hurtado. (2001). Una introduccion a los numeros p-adicos. Memorias XVIII encuentro de geometrıa y VI de aritmetica, 359 – 369. Disponível em: http://funes.uniandes.edu.co/5615/1/CarrilloUnaintroduccionGeometr%C3%ADa2008.PDF
VACCON. Tristan. (2015). Précision p-adique. (thésis de doctorat). Rennes: Université de Rennes. Disponível em: https://tel.archives-ouvertes.fr/tel-01205269v2/document
ALVES, Francisco, R. V. (2017). Fórmula de de moivre, ou de binet ou de lamé: demonstrações e generalidades sobre a sequência generalizada de fibonacci – SGF. Revista Brasileira de História da Matemática, v, 17, nº 33, 1 – 16.
AMICE, Ivete. (1975). Le nombres p-adique. Paris: Presses Universitaire. Disponível em: https://www-fourier.ujf-grenoble.fr/~panchish/GDL16/amice_les-nombres-p-adiques%20%5B2755599%5D.pdf
AÇIKGÖZ, Mehmet; ASLAN,Nurgül; Köşkeroğlu, Nurten e Aracı, Serkan. (2009). p-Adic Approach to Linear 2-Normed Spaces. Mathematica Moravica, v. 13, nº 2, 7 – 21. Disponível em: http://scindeks-clanci.ceon.rs/data/pdf/1450-5932/2009/1450-59320902007A.pdf
ADERBURG, Drew. (2002). A Mathematical Seduction. Math Horizons, 9:3, 12-15. Disponível em: https://web.williams.edu/Mathematics/eburger/BurgerMathHorizons.pdf
BELAIR, Luc. (2012). Panorama of p-adic model theory, Annales des Sciences Mathematiques du Quebec, nº 36, p. 43-75, 2012. Disponível em: http://www.logique.jussieu.fr/modnet/Publications/Introductory%20Notes%20and%20surveys/Belair.pdf
BOREVICH, Z. I.; SHAFAREVICH, I. R. (1966). Number Theory. New York: Academic Press.
BURGER, Edward, B. (2000). Exploring the number jungle: a journey into diophantine analysis. New York: Americal Mathematical Society.
CĂLIN, Mureşan Alexe. (2006). Non-Archimedian Fields. Topological Properties of Zp, Qp (p-adics Numbers). Matematică - Informatică – Fizică, v. 63, nº 2, 43 – 48. Disponível em: http://bmif.unde.ro/docs/20062/7%20MuresanA.pdf
CARVALHO, Maria Pires de; LOURENÇO, João Nuno P. (2015). Convergência de séries p-ádicas. Boletim da Sociedade Portuguesa de Matemática, 1 – 30, Disponível em: https://repositorio-aberto.up.pt/bitstream/10216/90725/2/104694.pdf
CHINEA, C. (2000). Matemática. ¿qué son los números p-adicos?. carlos s. chinea, octubr. Divulgación de la matemática en la red. 1 – 5. Disponível em: https://casanchi.com/casanchi_2000/19_padicos01.pdf
CORNELISSEN, Gunther; KATO, Fumiharu. (2005). The p-adic icosaedron. Notes in American Mathematical Association - AMS. v. 52, nº 7, 720 – 727. Disponível em: https://www.ams.org/notices/200507/fea-cornelissen.pdf
COHEN, Henri. (2007). Number Theory Volume I: Tools and Diophantine Equations. New York: Springer.
CORRY, Leo. (2004). Modern Algebra and the Rise of Mathematical Structures, Basel and Boston, Birkhäuser.
CUOCO, Albert. A. Visualizing the p-adic integers. American Mathematical Monthly. v. 98, nº 4, 355 – 364. Disponível em: https://www.jstor.org/stable/pdf/2323809.pdf?refreqid=excelsior%3Ada3401d345ac7e62ccac8f6378fb2cfc
CROMPTON, Catherine (2007) Some Geometry of the p-adic rationals. Rose-Hulman Undergraduate Mathematics Journal: v. 8 : Iss. 1 , Article 2. 1 – 13. Disponível em: https://scholar.rose-hulman.edu/cgi/viewcontent.cgi?referer=https://www.google.com.br/&httpsredir=1&article=1183&context=rhumj
DELAHAYE. Jean-Paul. (2001). Les nombres infinit vers la gauche. Pour la Science, nº 279, 100 – 104. Disponível em: http://www.lifl.fr/~jdelahay/pls/081.pdf
EHRLICH. Philip. (2006). The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Emergence of non-Archimedean Systems of Magnitudes. The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Archive History in Exact Sciences. V. 60, 1 – 121. Disponível em: http://prima.lnu.edu.ua/faculty/mechmat/Departments/MFAUKR/attachments/erlich.pdf
FERREIRA, Jamil. (2010). A construção dos números. Textos Universitários. Rio de Janeiro: SBM.
GOLDBLATT, Robert. (1998). Lectures on the Hyprreeals numbers: an introduction to non standard analisys. New York: Springer.
GOUVÊA, F. Q. (1997). p-adic numbers. New York: Springer.
GUSMÃO, Italo, B. (2016). Números p-ádicos. Dissertação de Mestrado Profissional PROFMAT, João Pessoa: UFPB.. Disponível em: https://repositorio.ufpb.br/jspui/bitstream/tede/9337/2/arquivototal.pdf
GAUTSCHI, Walter. Alessandro M. (2002). Ostrowski (1893–1986): la sua vita e le opere. Boll. Docenti Matem. v. 45, 9–19. Disponível em: https://www.cs.purdue.edu/homes/wxg/AMOital.pdf
HASSE, Helmut. (1980). Number Theory. Springer: New York.
HEFEZ, Abramo. (2013). Um curso de Àlgebra, v. 1, Rio de Janeiro: SBM.
HOLLY, Jan E. (2001). Pictures of Ultrametric Spaces, the p-adic Numbers, and Valued Fields. The mathematical association of America. October, 721 – 728. Disponível em: https://www.colby.edu/math/faculty/Faculty_files/hollydir/Holly01.pdf
HUAMAN, Ronald M. (2015). Raıces p-adicas de la unidad. (Tesis de Maestrıa). Pontificia Universidad del Perú: Lima. Disponível em: http://tesis.pucp.edu.pe/repositorio/bitstream/handle/123456789/6415/MAS_HUAMAN_RONALD_PADICAS.pdf;sequence=1
LAGES, Elon. (2010). Curso de Análise, v. 1. Rio de Janeiro: SBM.
LAPIDUS, Michel L.; HUNG. Lu. (2011). The Geometry of p-Adic Fractal Strings: A Comparative Survey. Contemporary Mathematics, v. 551, 163 – 206. Disponível em: http://www.math.ucr.edu/~lapidus/papers/ContMath/GeometrypAdicStringsSurvey10893.pdf
LAPIDUS, Michel L.; HUNG. Lu. (2008). Nonarchimedean Cantor set and string. Journal of Fixed Point Theory and Applications. v. 4, p. 1 – 10. Disponível em: https://pdfs.semanticscholar.org/6dee/45208234f48fe156d174d4ad56b17363d2ef.pdf
LEGUAY, Mathieu; HENRI, Joseph. (1992). Distance p-adique : une distance qui n'est pas habituelle. MATh.en.JEANS” au Palais de la Découverte. 33 – 36. Disponível em: http://mathenjeans.free.fr/amej/edition/actes/actespdf/92033036.pdf
MARCOS, José. E. (2006). The algebraic closure of a p-adic number field is a complete topological field. Mathematica Slovaca,v. 56, No. 3, p. 317—331. Disponível em: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.1010.7259&rep=rep1&type=pdf
MASIAS, Henry Zorrilla. (2011). Complecion no arquimedeana. Pro Mathematica, v. 25, 49-50. Disponível em: http://revistas.pucp.edu.pe/index.php/promathematica/article/viewFile/2666/2610
NARICI, Lawrence; BECKENSTEIN, Edward. (1981). Strange Terrain--Nonarchimedean Spaces. The American Mathematical Monthly, Vol. 88, No. 9 (Nov., 1981), pp. 667-676. Disponível em: https://www.jstor.org/stable/pdf/2320670.pdf
OSTROWSKI, A. (1916). Uber einige L¨osungen der Funktionalgleichung ¨ φ(x)φ(y) = φ(xy)”, Acta Mathematica (2nd ed.), nº 41, no. 1, 271–284. Disponível em: https://projecteuclid.org/euclid.acta/1485887472
KATO, Kazuya; KUROKAWA, Nobushige; SAITO, Takeshi, (2000). Number theory 1: Fermat’s dream, Translations of Mathematical Monographs, vol. 186, American Mathematical Society, Providence, RI.
KATOK, S. (2007). p-adic analysis compared with real. Student mathematical library, American Mathematical Soc.
KOBLITZ, Neal. (1984). p-adic Numbers, p-adic Analysis, and Zeta- Functions. New York: Springer.
KHRENNIKOV, Andrei; KOTOVICHM, Nikolay. (2017). Image Segmentation with the Aid of the p-Adic Metrics. In: TONI, Bourama. New Trends and Advanced Methods in Interdisciplinary Mathematical Sciences. 143 – 155.
KUMAR, Ashish; RANI, Mamta; CHUGH, Renu. (2013). New 5-adic Cantor sets and fractal string. SpringerPlus, 1 – 7. Disponível em: https://link.springer.com/content/pdf/10.1186%2F2193-1801-2-654.pdf
LEWIS, Robert Y. (2019). A formal proof of Hensel’s lemma over the p-adic integers. CPP’19, January p. 14–15, Lisbon, PT. Disponível em: http://robertylewis.com/padics/padics.pdf
NATARAJAN, P N; RANGANATHAN, K. N. (2000). A Geometry in which all Triangles are Isosceles An Introduction to Non-Archimedean Analysis. Resonance, October, 32 – 42. Disponível em: https://www.ias.ac.in/article/fulltext/reso/005/10/0032-0042
NEUKIRCH, J. (1990). The p-Adic numbers. EBBINGHAUS, H.-D. Numbers. New York: Springer. 155 – 179.
OLIVEIRA, Graciano. (2009). O corpo dos p-ádicos. Gazeta Matemática, Dezembro, 7 – 18. Disponível em: http://gazeta.spm.pt/getArtigo?gid=258
ORBEGOSO, Jorge Luis Rojas. Completitud y clausura algebraica de campos P-ádicos. Universidad nacional mayor de san marcos. Perú: Lima, 2016. Disponível em: http://cybertesis.unmsm.edu.pe/bitstream/handle/cybertesis/6315/Rojas_oj.pdf?sequence=1
PITANEN, Matti. (2015). How Imagination Could Be Realized p-Adically?. Journal of Consciousness Exploration & Research, v 6, nº 6, 354 – 356. Disponível em: https://jcer.com/index.php/jcj/article/viewFile/468/488
REDDY, B. Surender; SHANKARAIAH, D. (2013). On i-cauchy sequences in p-adic linear 2-normed spaces. International Journal of Pure and Applied Mathematics. v. 89, nº 4, 483 – 496. Disponivel em: https://ijpam.eu/contents/2013-89-4/4/4.pdf
RIBENBOIM, Paulo. (1999). The Theory of Classical Valuations. New York: Springer.
ROBERT, Alain. (1996). Qu´est-qe que le nombres p- ádique? Societé des enseignants neuchâtelois des Sciences. Bulletin, setembre, 5 – 12. Disponível em: http://www.sens-neuchatel.ch/bulletin/anciens-no-pdf/BULL18.PDF
ROZIKOV, U. A. (2013). What are p-Adic Numbers? What are They Used for?. Asia Pacific Mathematics Newsletter. v. 3, nº 4, 1 – 6, October. Disponível em: http://www.asiapacific-mathnews.com/03/0304/0001_0006.pdf
SCHIKHOF W. H. (1984). Ultrametric Calculus: An introduction to p-adic Analysis. Cambridge: University Press.
SCHLICHENMAIER, Martin. (2007). An Introduction to Riemann Surfaces, Algebraic Curves and Moduli Spaces. Springer: New York.
SILVERMANM, Joseph H. (2013). What is the p-adic Mandelbrot Set?. Notices of the AMS, setember, v. 60, nº 8, 1048 – 1050. Disponível em: http://www.ams.org/notices/201308/201308-full-issue.pdf
STEUDING, Jorn. (2002). The world of p-adic numbers and p-adic functions. Faculty of Physics and Mathematics. nº 5, 90 – 107. Disponível em: http://siauliaims.su.lt/pdfai/2002/STEUD-02.pdf
TEITELBAUM. Jeremy. (1995). The Geometry of p-adic Symmetric Spaces. Notice in American Mathematical Monthly. v. 42, nº 10, 1120 – 1126. Disponível em: https://www.ams.org/notices/199510/teitelbaum.pdf
TELLER, Jacek. (2012). Newton polygons on p-adic number fields. (dissertation in Arts and Mathematics). East Caroline University. Disponível em: http://thescholarship.ecu.edu/bitstream/handle/10342/3848/Teller_ecu_0600M_10676.pdf?sequence=1
TORRES, Sergio Carrillo; AMAYA, Carlos Hurtado. (2001). Una introduccion a los numeros p-adicos. Memorias XVIII encuentro de geometrıa y VI de aritmetica, 359 – 369. Disponível em: http://funes.uniandes.edu.co/5615/1/CarrilloUnaintroduccionGeometr%C3%ADa2008.PDF
VACCON. Tristan. (2015). Précision p-adique. (thésis de doctorat). Rennes: Université de Rennes. Disponível em: https://tel.archives-ouvertes.fr/tel-01205269v2/document
AMICE, Ivete. (1975). Le nombres p-adique. Paris: Presses Universitaire. Disponível em: https://www-fourier.ujf-grenoble.fr/~panchish/GDL16/amice_les-nombres-p-adiques%20%5B2755599%5D.pdf
AÇIKGÖZ, Mehmet; ASLAN,Nurgül; Köşkeroğlu, Nurten e Aracı, Serkan. (2009). p-Adic Approach to Linear 2-Normed Spaces. Mathematica Moravica, v. 13, nº 2, 7 – 21. Disponível em: http://scindeks-clanci.ceon.rs/data/pdf/1450-5932/2009/1450-59320902007A.pdf
ADERBURG, Drew. (2002). A Mathematical Seduction. Math Horizons, 9:3, 12-15. Disponível em: https://web.williams.edu/Mathematics/eburger/BurgerMathHorizons.pdf
BELAIR, Luc. (2012). Panorama of p-adic model theory, Annales des Sciences Mathematiques du Quebec, nº 36, p. 43-75, 2012. Disponível em: http://www.logique.jussieu.fr/modnet/Publications/Introductory%20Notes%20and%20surveys/Belair.pdf
BOREVICH, Z. I.; SHAFAREVICH, I. R. (1966). Number Theory. New York: Academic Press.
BURGER, Edward, B. (2000). Exploring the number jungle: a journey into diophantine analysis. New York: Americal Mathematical Society.
CĂLIN, Mureşan Alexe. (2006). Non-Archimedian Fields. Topological Properties of Zp, Qp (p-adics Numbers). Matematică - Informatică – Fizică, v. 63, nº 2, 43 – 48. Disponível em: http://bmif.unde.ro/docs/20062/7%20MuresanA.pdf
CARVALHO, Maria Pires de; LOURENÇO, João Nuno P. (2015). Convergência de séries p-ádicas. Boletim da Sociedade Portuguesa de Matemática, 1 – 30, Disponível em: https://repositorio-aberto.up.pt/bitstream/10216/90725/2/104694.pdf
CHINEA, C. (2000). Matemática. ¿qué son los números p-adicos?. carlos s. chinea, octubr. Divulgación de la matemática en la red. 1 – 5. Disponível em: https://casanchi.com/casanchi_2000/19_padicos01.pdf
CORNELISSEN, Gunther; KATO, Fumiharu. (2005). The p-adic icosaedron. Notes in American Mathematical Association - AMS. v. 52, nº 7, 720 – 727. Disponível em: https://www.ams.org/notices/200507/fea-cornelissen.pdf
COHEN, Henri. (2007). Number Theory Volume I: Tools and Diophantine Equations. New York: Springer.
CORRY, Leo. (2004). Modern Algebra and the Rise of Mathematical Structures, Basel and Boston, Birkhäuser.
CUOCO, Albert. A. Visualizing the p-adic integers. American Mathematical Monthly. v. 98, nº 4, 355 – 364. Disponível em: https://www.jstor.org/stable/pdf/2323809.pdf?refreqid=excelsior%3Ada3401d345ac7e62ccac8f6378fb2cfc
CROMPTON, Catherine (2007) Some Geometry of the p-adic rationals. Rose-Hulman Undergraduate Mathematics Journal: v. 8 : Iss. 1 , Article 2. 1 – 13. Disponível em: https://scholar.rose-hulman.edu/cgi/viewcontent.cgi?referer=https://www.google.com.br/&httpsredir=1&article=1183&context=rhumj
DELAHAYE. Jean-Paul. (2001). Les nombres infinit vers la gauche. Pour la Science, nº 279, 100 – 104. Disponível em: http://www.lifl.fr/~jdelahay/pls/081.pdf
EHRLICH. Philip. (2006). The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Emergence of non-Archimedean Systems of Magnitudes. The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Archive History in Exact Sciences. V. 60, 1 – 121. Disponível em: http://prima.lnu.edu.ua/faculty/mechmat/Departments/MFAUKR/attachments/erlich.pdf
FERREIRA, Jamil. (2010). A construção dos números. Textos Universitários. Rio de Janeiro: SBM.
GOLDBLATT, Robert. (1998). Lectures on the Hyprreeals numbers: an introduction to non standard analisys. New York: Springer.
GOUVÊA, F. Q. (1997). p-adic numbers. New York: Springer.
GUSMÃO, Italo, B. (2016). Números p-ádicos. Dissertação de Mestrado Profissional PROFMAT, João Pessoa: UFPB.. Disponível em: https://repositorio.ufpb.br/jspui/bitstream/tede/9337/2/arquivototal.pdf
GAUTSCHI, Walter. Alessandro M. (2002). Ostrowski (1893–1986): la sua vita e le opere. Boll. Docenti Matem. v. 45, 9–19. Disponível em: https://www.cs.purdue.edu/homes/wxg/AMOital.pdf
HASSE, Helmut. (1980). Number Theory. Springer: New York.
HEFEZ, Abramo. (2013). Um curso de Àlgebra, v. 1, Rio de Janeiro: SBM.
HOLLY, Jan E. (2001). Pictures of Ultrametric Spaces, the p-adic Numbers, and Valued Fields. The mathematical association of America. October, 721 – 728. Disponível em: https://www.colby.edu/math/faculty/Faculty_files/hollydir/Holly01.pdf
HUAMAN, Ronald M. (2015). Raıces p-adicas de la unidad. (Tesis de Maestrıa). Pontificia Universidad del Perú: Lima. Disponível em: http://tesis.pucp.edu.pe/repositorio/bitstream/handle/123456789/6415/MAS_HUAMAN_RONALD_PADICAS.pdf;sequence=1
LAGES, Elon. (2010). Curso de Análise, v. 1. Rio de Janeiro: SBM.
LAPIDUS, Michel L.; HUNG. Lu. (2011). The Geometry of p-Adic Fractal Strings: A Comparative Survey. Contemporary Mathematics, v. 551, 163 – 206. Disponível em: http://www.math.ucr.edu/~lapidus/papers/ContMath/GeometrypAdicStringsSurvey10893.pdf
LAPIDUS, Michel L.; HUNG. Lu. (2008). Nonarchimedean Cantor set and string. Journal of Fixed Point Theory and Applications. v. 4, p. 1 – 10. Disponível em: https://pdfs.semanticscholar.org/6dee/45208234f48fe156d174d4ad56b17363d2ef.pdf
LEGUAY, Mathieu; HENRI, Joseph. (1992). Distance p-adique : une distance qui n'est pas habituelle. MATh.en.JEANS” au Palais de la Découverte. 33 – 36. Disponível em: http://mathenjeans.free.fr/amej/edition/actes/actespdf/92033036.pdf
MARCOS, José. E. (2006). The algebraic closure of a p-adic number field is a complete topological field. Mathematica Slovaca,v. 56, No. 3, p. 317—331. Disponível em: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.1010.7259&rep=rep1&type=pdf
MASIAS, Henry Zorrilla. (2011). Complecion no arquimedeana. Pro Mathematica, v. 25, 49-50. Disponível em: http://revistas.pucp.edu.pe/index.php/promathematica/article/viewFile/2666/2610
NARICI, Lawrence; BECKENSTEIN, Edward. (1981). Strange Terrain--Nonarchimedean Spaces. The American Mathematical Monthly, Vol. 88, No. 9 (Nov., 1981), pp. 667-676. Disponível em: https://www.jstor.org/stable/pdf/2320670.pdf
OSTROWSKI, A. (1916). Uber einige L¨osungen der Funktionalgleichung ¨ φ(x)φ(y) = φ(xy)”, Acta Mathematica (2nd ed.), nº 41, no. 1, 271–284. Disponível em: https://projecteuclid.org/euclid.acta/1485887472
KATO, Kazuya; KUROKAWA, Nobushige; SAITO, Takeshi, (2000). Number theory 1: Fermat’s dream, Translations of Mathematical Monographs, vol. 186, American Mathematical Society, Providence, RI.
KATOK, S. (2007). p-adic analysis compared with real. Student mathematical library, American Mathematical Soc.
KOBLITZ, Neal. (1984). p-adic Numbers, p-adic Analysis, and Zeta- Functions. New York: Springer.
KHRENNIKOV, Andrei; KOTOVICHM, Nikolay. (2017). Image Segmentation with the Aid of the p-Adic Metrics. In: TONI, Bourama. New Trends and Advanced Methods in Interdisciplinary Mathematical Sciences. 143 – 155.
KUMAR, Ashish; RANI, Mamta; CHUGH, Renu. (2013). New 5-adic Cantor sets and fractal string. SpringerPlus, 1 – 7. Disponível em: https://link.springer.com/content/pdf/10.1186%2F2193-1801-2-654.pdf
LEWIS, Robert Y. (2019). A formal proof of Hensel’s lemma over the p-adic integers. CPP’19, January p. 14–15, Lisbon, PT. Disponível em: http://robertylewis.com/padics/padics.pdf
NATARAJAN, P N; RANGANATHAN, K. N. (2000). A Geometry in which all Triangles are Isosceles An Introduction to Non-Archimedean Analysis. Resonance, October, 32 – 42. Disponível em: https://www.ias.ac.in/article/fulltext/reso/005/10/0032-0042
NEUKIRCH, J. (1990). The p-Adic numbers. EBBINGHAUS, H.-D. Numbers. New York: Springer. 155 – 179.
OLIVEIRA, Graciano. (2009). O corpo dos p-ádicos. Gazeta Matemática, Dezembro, 7 – 18. Disponível em: http://gazeta.spm.pt/getArtigo?gid=258
ORBEGOSO, Jorge Luis Rojas. Completitud y clausura algebraica de campos P-ádicos. Universidad nacional mayor de san marcos. Perú: Lima, 2016. Disponível em: http://cybertesis.unmsm.edu.pe/bitstream/handle/cybertesis/6315/Rojas_oj.pdf?sequence=1
PITANEN, Matti. (2015). How Imagination Could Be Realized p-Adically?. Journal of Consciousness Exploration & Research, v 6, nº 6, 354 – 356. Disponível em: https://jcer.com/index.php/jcj/article/viewFile/468/488
REDDY, B. Surender; SHANKARAIAH, D. (2013). On i-cauchy sequences in p-adic linear 2-normed spaces. International Journal of Pure and Applied Mathematics. v. 89, nº 4, 483 – 496. Disponivel em: https://ijpam.eu/contents/2013-89-4/4/4.pdf
RIBENBOIM, Paulo. (1999). The Theory of Classical Valuations. New York: Springer.
ROBERT, Alain. (1996). Qu´est-qe que le nombres p- ádique? Societé des enseignants neuchâtelois des Sciences. Bulletin, setembre, 5 – 12. Disponível em: http://www.sens-neuchatel.ch/bulletin/anciens-no-pdf/BULL18.PDF
ROZIKOV, U. A. (2013). What are p-Adic Numbers? What are They Used for?. Asia Pacific Mathematics Newsletter. v. 3, nº 4, 1 – 6, October. Disponível em: http://www.asiapacific-mathnews.com/03/0304/0001_0006.pdf
SCHIKHOF W. H. (1984). Ultrametric Calculus: An introduction to p-adic Analysis. Cambridge: University Press.
SCHLICHENMAIER, Martin. (2007). An Introduction to Riemann Surfaces, Algebraic Curves and Moduli Spaces. Springer: New York.
SILVERMANM, Joseph H. (2013). What is the p-adic Mandelbrot Set?. Notices of the AMS, setember, v. 60, nº 8, 1048 – 1050. Disponível em: http://www.ams.org/notices/201308/201308-full-issue.pdf
STEUDING, Jorn. (2002). The world of p-adic numbers and p-adic functions. Faculty of Physics and Mathematics. nº 5, 90 – 107. Disponível em: http://siauliaims.su.lt/pdfai/2002/STEUD-02.pdf
TEITELBAUM. Jeremy. (1995). The Geometry of p-adic Symmetric Spaces. Notice in American Mathematical Monthly. v. 42, nº 10, 1120 – 1126. Disponível em: https://www.ams.org/notices/199510/teitelbaum.pdf
TELLER, Jacek. (2012). Newton polygons on p-adic number fields. (dissertation in Arts and Mathematics). East Caroline University. Disponível em: http://thescholarship.ecu.edu/bitstream/handle/10342/3848/Teller_ecu_0600M_10676.pdf?sequence=1
TORRES, Sergio Carrillo; AMAYA, Carlos Hurtado. (2001). Una introduccion a los numeros p-adicos. Memorias XVIII encuentro de geometrıa y VI de aritmetica, 359 – 369. Disponível em: http://funes.uniandes.edu.co/5615/1/CarrilloUnaintroduccionGeometr%C3%ADa2008.PDF
VACCON. Tristan. (2015). Précision p-adique. (thésis de doctorat). Rennes: Université de Rennes. Disponível em: https://tel.archives-ouvertes.fr/tel-01205269v2/document
ALVES, Francisco, R. V. (2017). Fórmula de de moivre, ou de binet ou de lamé: demonstrações e generalidades sobre a sequência generalizada de fibonacci – SGF. Revista Brasileira de História da Matemática, v, 17, nº 33, 1 – 16.
AMICE, Ivete. (1975). Le nombres p-adique. Paris: Presses Universitaire. Disponível em: https://www-fourier.ujf-grenoble.fr/~panchish/GDL16/amice_les-nombres-p-adiques%20%5B2755599%5D.pdf
AÇIKGÖZ, Mehmet; ASLAN,Nurgül; Köşkeroğlu, Nurten e Aracı, Serkan. (2009). p-Adic Approach to Linear 2-Normed Spaces. Mathematica Moravica, v. 13, nº 2, 7 – 21. Disponível em: http://scindeks-clanci.ceon.rs/data/pdf/1450-5932/2009/1450-59320902007A.pdf
ADERBURG, Drew. (2002). A Mathematical Seduction. Math Horizons, 9:3, 12-15. Disponível em: https://web.williams.edu/Mathematics/eburger/BurgerMathHorizons.pdf
BELAIR, Luc. (2012). Panorama of p-adic model theory, Annales des Sciences Mathematiques du Quebec, nº 36, p. 43-75, 2012. Disponível em: http://www.logique.jussieu.fr/modnet/Publications/Introductory%20Notes%20and%20surveys/Belair.pdf
BOREVICH, Z. I.; SHAFAREVICH, I. R. (1966). Number Theory. New York: Academic Press.
BURGER, Edward, B. (2000). Exploring the number jungle: a journey into diophantine analysis. New York: Americal Mathematical Society.
CĂLIN, Mureşan Alexe. (2006). Non-Archimedian Fields. Topological Properties of Zp, Qp (p-adics Numbers). Matematică - Informatică – Fizică, v. 63, nº 2, 43 – 48. Disponível em: http://bmif.unde.ro/docs/20062/7%20MuresanA.pdf
CARVALHO, Maria Pires de; LOURENÇO, João Nuno P. (2015). Convergência de séries p-ádicas. Boletim da Sociedade Portuguesa de Matemática, 1 – 30, Disponível em: https://repositorio-aberto.up.pt/bitstream/10216/90725/2/104694.pdf
CHINEA, C. (2000). Matemática. ¿qué son los números p-adicos?. carlos s. chinea, octubr. Divulgación de la matemática en la red. 1 – 5. Disponível em: https://casanchi.com/casanchi_2000/19_padicos01.pdf
CORNELISSEN, Gunther; KATO, Fumiharu. (2005). The p-adic icosaedron. Notes in American Mathematical Association - AMS. v. 52, nº 7, 720 – 727. Disponível em: https://www.ams.org/notices/200507/fea-cornelissen.pdf
COHEN, Henri. (2007). Number Theory Volume I: Tools and Diophantine Equations. New York: Springer.
CORRY, Leo. (2004). Modern Algebra and the Rise of Mathematical Structures, Basel and Boston, Birkhäuser.
CUOCO, Albert. A. Visualizing the p-adic integers. American Mathematical Monthly. v. 98, nº 4, 355 – 364. Disponível em: https://www.jstor.org/stable/pdf/2323809.pdf?refreqid=excelsior%3Ada3401d345ac7e62ccac8f6378fb2cfc
CROMPTON, Catherine (2007) Some Geometry of the p-adic rationals. Rose-Hulman Undergraduate Mathematics Journal: v. 8 : Iss. 1 , Article 2. 1 – 13. Disponível em: https://scholar.rose-hulman.edu/cgi/viewcontent.cgi?referer=https://www.google.com.br/&httpsredir=1&article=1183&context=rhumj
DELAHAYE. Jean-Paul. (2001). Les nombres infinit vers la gauche. Pour la Science, nº 279, 100 – 104. Disponível em: http://www.lifl.fr/~jdelahay/pls/081.pdf
EHRLICH. Philip. (2006). The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Emergence of non-Archimedean Systems of Magnitudes. The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Archive History in Exact Sciences. V. 60, 1 – 121. Disponível em: http://prima.lnu.edu.ua/faculty/mechmat/Departments/MFAUKR/attachments/erlich.pdf
FERREIRA, Jamil. (2010). A construção dos números. Textos Universitários. Rio de Janeiro: SBM.
GOLDBLATT, Robert. (1998). Lectures on the Hyprreeals numbers: an introduction to non standard analisys. New York: Springer.
GOUVÊA, F. Q. (1997). p-adic numbers. New York: Springer.
GUSMÃO, Italo, B. (2016). Números p-ádicos. Dissertação de Mestrado Profissional PROFMAT, João Pessoa: UFPB.. Disponível em: https://repositorio.ufpb.br/jspui/bitstream/tede/9337/2/arquivototal.pdf
GAUTSCHI, Walter. Alessandro M. (2002). Ostrowski (1893–1986): la sua vita e le opere. Boll. Docenti Matem. v. 45, 9–19. Disponível em: https://www.cs.purdue.edu/homes/wxg/AMOital.pdf
HASSE, Helmut. (1980). Number Theory. Springer: New York.
HEFEZ, Abramo. (2013). Um curso de Àlgebra, v. 1, Rio de Janeiro: SBM.
HOLLY, Jan E. (2001). Pictures of Ultrametric Spaces, the p-adic Numbers, and Valued Fields. The mathematical association of America. October, 721 – 728. Disponível em: https://www.colby.edu/math/faculty/Faculty_files/hollydir/Holly01.pdf
HUAMAN, Ronald M. (2015). Raıces p-adicas de la unidad. (Tesis de Maestrıa). Pontificia Universidad del Perú: Lima. Disponível em: http://tesis.pucp.edu.pe/repositorio/bitstream/handle/123456789/6415/MAS_HUAMAN_RONALD_PADICAS.pdf;sequence=1
LAGES, Elon. (2010). Curso de Análise, v. 1. Rio de Janeiro: SBM.
LAPIDUS, Michel L.; HUNG. Lu. (2011). The Geometry of p-Adic Fractal Strings: A Comparative Survey. Contemporary Mathematics, v. 551, 163 – 206. Disponível em: http://www.math.ucr.edu/~lapidus/papers/ContMath/GeometrypAdicStringsSurvey10893.pdf
LAPIDUS, Michel L.; HUNG. Lu. (2008). Nonarchimedean Cantor set and string. Journal of Fixed Point Theory and Applications. v. 4, p. 1 – 10. Disponível em: https://pdfs.semanticscholar.org/6dee/45208234f48fe156d174d4ad56b17363d2ef.pdf
LEGUAY, Mathieu; HENRI, Joseph. (1992). Distance p-adique : une distance qui n'est pas habituelle. MATh.en.JEANS” au Palais de la Découverte. 33 – 36. Disponível em: http://mathenjeans.free.fr/amej/edition/actes/actespdf/92033036.pdf
MARCOS, José. E. (2006). The algebraic closure of a p-adic number field is a complete topological field. Mathematica Slovaca,v. 56, No. 3, p. 317—331. Disponível em: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.1010.7259&rep=rep1&type=pdf
MASIAS, Henry Zorrilla. (2011). Complecion no arquimedeana. Pro Mathematica, v. 25, 49-50. Disponível em: http://revistas.pucp.edu.pe/index.php/promathematica/article/viewFile/2666/2610
NARICI, Lawrence; BECKENSTEIN, Edward. (1981). Strange Terrain--Nonarchimedean Spaces. The American Mathematical Monthly, Vol. 88, No. 9 (Nov., 1981), pp. 667-676. Disponível em: https://www.jstor.org/stable/pdf/2320670.pdf
OSTROWSKI, A. (1916). Uber einige L¨osungen der Funktionalgleichung ¨ φ(x)φ(y) = φ(xy)”, Acta Mathematica (2nd ed.), nº 41, no. 1, 271–284. Disponível em: https://projecteuclid.org/euclid.acta/1485887472
KATO, Kazuya; KUROKAWA, Nobushige; SAITO, Takeshi, (2000). Number theory 1: Fermat’s dream, Translations of Mathematical Monographs, vol. 186, American Mathematical Society, Providence, RI.
KATOK, S. (2007). p-adic analysis compared with real. Student mathematical library, American Mathematical Soc.
KOBLITZ, Neal. (1984). p-adic Numbers, p-adic Analysis, and Zeta- Functions. New York: Springer.
KHRENNIKOV, Andrei; KOTOVICHM, Nikolay. (2017). Image Segmentation with the Aid of the p-Adic Metrics. In: TONI, Bourama. New Trends and Advanced Methods in Interdisciplinary Mathematical Sciences. 143 – 155.
KUMAR, Ashish; RANI, Mamta; CHUGH, Renu. (2013). New 5-adic Cantor sets and fractal string. SpringerPlus, 1 – 7. Disponível em: https://link.springer.com/content/pdf/10.1186%2F2193-1801-2-654.pdf
LEWIS, Robert Y. (2019). A formal proof of Hensel’s lemma over the p-adic integers. CPP’19, January p. 14–15, Lisbon, PT. Disponível em: http://robertylewis.com/padics/padics.pdf
NATARAJAN, P N; RANGANATHAN, K. N. (2000). A Geometry in which all Triangles are Isosceles An Introduction to Non-Archimedean Analysis. Resonance, October, 32 – 42. Disponível em: https://www.ias.ac.in/article/fulltext/reso/005/10/0032-0042
NEUKIRCH, J. (1990). The p-Adic numbers. EBBINGHAUS, H.-D. Numbers. New York: Springer. 155 – 179.
OLIVEIRA, Graciano. (2009). O corpo dos p-ádicos. Gazeta Matemática, Dezembro, 7 – 18. Disponível em: http://gazeta.spm.pt/getArtigo?gid=258
ORBEGOSO, Jorge Luis Rojas. Completitud y clausura algebraica de campos P-ádicos. Universidad nacional mayor de san marcos. Perú: Lima, 2016. Disponível em: http://cybertesis.unmsm.edu.pe/bitstream/handle/cybertesis/6315/Rojas_oj.pdf?sequence=1
PITANEN, Matti. (2015). How Imagination Could Be Realized p-Adically?. Journal of Consciousness Exploration & Research, v 6, nº 6, 354 – 356. Disponível em: https://jcer.com/index.php/jcj/article/viewFile/468/488
REDDY, B. Surender; SHANKARAIAH, D. (2013). On i-cauchy sequences in p-adic linear 2-normed spaces. International Journal of Pure and Applied Mathematics. v. 89, nº 4, 483 – 496. Disponivel em: https://ijpam.eu/contents/2013-89-4/4/4.pdf
RIBENBOIM, Paulo. (1999). The Theory of Classical Valuations. New York: Springer.
ROBERT, Alain. (1996). Qu´est-qe que le nombres p- ádique? Societé des enseignants neuchâtelois des Sciences. Bulletin, setembre, 5 – 12. Disponível em: http://www.sens-neuchatel.ch/bulletin/anciens-no-pdf/BULL18.PDF
ROZIKOV, U. A. (2013). What are p-Adic Numbers? What are They Used for?. Asia Pacific Mathematics Newsletter. v. 3, nº 4, 1 – 6, October. Disponível em: http://www.asiapacific-mathnews.com/03/0304/0001_0006.pdf
SCHIKHOF W. H. (1984). Ultrametric Calculus: An introduction to p-adic Analysis. Cambridge: University Press.
SCHLICHENMAIER, Martin. (2007). An Introduction to Riemann Surfaces, Algebraic Curves and Moduli Spaces. Springer: New York.
SILVERMANM, Joseph H. (2013). What is the p-adic Mandelbrot Set?. Notices of the AMS, setember, v. 60, nº 8, 1048 – 1050. Disponível em: http://www.ams.org/notices/201308/201308-full-issue.pdf
STEUDING, Jorn. (2002). The world of p-adic numbers and p-adic functions. Faculty of Physics and Mathematics. nº 5, 90 – 107. Disponível em: http://siauliaims.su.lt/pdfai/2002/STEUD-02.pdf
TEITELBAUM. Jeremy. (1995). The Geometry of p-adic Symmetric Spaces. Notice in American Mathematical Monthly. v. 42, nº 10, 1120 – 1126. Disponível em: https://www.ams.org/notices/199510/teitelbaum.pdf
TELLER, Jacek. (2012). Newton polygons on p-adic number fields. (dissertation in Arts and Mathematics). East Caroline University. Disponível em: http://thescholarship.ecu.edu/bitstream/handle/10342/3848/Teller_ecu_0600M_10676.pdf?sequence=1
TORRES, Sergio Carrillo; AMAYA, Carlos Hurtado. (2001). Una introduccion a los numeros p-adicos. Memorias XVIII encuentro de geometrıa y VI de aritmetica, 359 – 369. Disponível em: http://funes.uniandes.edu.co/5615/1/CarrilloUnaintroduccionGeometr%C3%ADa2008.PDF
VACCON. Tristan. (2015). Précision p-adique. (thésis de doctorat). Rennes: Université de Rennes. Disponível em: https://tel.archives-ouvertes.fr/tel-01205269v2/document
ALVES, Francisco, R. V. (2017). Fórmula de de moivre, ou de binet ou de lamé: demonstrações e generalidades sobre a sequência generalizada de fibonacci – SGF. Revista Brasileira de História da Matemática, v, 17, nº 33, 1 – 16.
AMICE, Ivete. (1975). Le nombres p-adique. Paris: Presses Universitaire. Disponível em: https://www-fourier.ujf-grenoble.fr/~panchish/GDL16/amice_les-nombres-p-adiques%20%5B2755599%5D.pdf
AÇIKGÖZ, Mehmet; ASLAN,Nurgül; Köşkeroğlu, Nurten e Aracı, Serkan. (2009). p-Adic Approach to Linear 2-Normed Spaces. Mathematica Moravica, v. 13, nº 2, 7 – 21. Disponível em: http://scindeks-clanci.ceon.rs/data/pdf/1450-5932/2009/1450-59320902007A.pdf
ADERBURG, Drew. (2002). A Mathematical Seduction. Math Horizons, 9:3, 12-15. Disponível em: https://web.williams.edu/Mathematics/eburger/BurgerMathHorizons.pdf
BELAIR, Luc. (2012). Panorama of p-adic model theory, Annales des Sciences Mathematiques du Quebec, nº 36, p. 43-75, 2012. Disponível em: http://www.logique.jussieu.fr/modnet/Publications/Introductory%20Notes%20and%20surveys/Belair.pdf
BOREVICH, Z. I.; SHAFAREVICH, I. R. (1966). Number Theory. New York: Academic Press.
BURGER, Edward, B. (2000). Exploring the number jungle: a journey into diophantine analysis. New York: Americal Mathematical Society.
CĂLIN, Mureşan Alexe. (2006). Non-Archimedian Fields. Topological Properties of Zp, Qp (p-adics Numbers). Matematică - Informatică – Fizică, v. 63, nº 2, 43 – 48. Disponível em: http://bmif.unde.ro/docs/20062/7%20MuresanA.pdf
CARVALHO, Maria Pires de; LOURENÇO, João Nuno P. (2015). Convergência de séries p-ádicas. Boletim da Sociedade Portuguesa de Matemática, 1 – 30, Disponível em: https://repositorio-aberto.up.pt/bitstream/10216/90725/2/104694.pdf
CHINEA, C. (2000). Matemática. ¿qué son los números p-adicos?. carlos s. chinea, octubr. Divulgación de la matemática en la red. 1 – 5. Disponível em: https://casanchi.com/casanchi_2000/19_padicos01.pdf
CORNELISSEN, Gunther; KATO, Fumiharu. (2005). The p-adic icosaedron. Notes in American Mathematical Association - AMS. v. 52, nº 7, 720 – 727. Disponível em: https://www.ams.org/notices/200507/fea-cornelissen.pdf
COHEN, Henri. (2007). Number Theory Volume I: Tools and Diophantine Equations. New York: Springer.
CORRY, Leo. (2004). Modern Algebra and the Rise of Mathematical Structures, Basel and Boston, Birkhäuser.
CUOCO, Albert. A. Visualizing the p-adic integers. American Mathematical Monthly. v. 98, nº 4, 355 – 364. Disponível em: https://www.jstor.org/stable/pdf/2323809.pdf?refreqid=excelsior%3Ada3401d345ac7e62ccac8f6378fb2cfc
CROMPTON, Catherine (2007) Some Geometry of the p-adic rationals. Rose-Hulman Undergraduate Mathematics Journal: v. 8 : Iss. 1 , Article 2. 1 – 13. Disponível em: https://scholar.rose-hulman.edu/cgi/viewcontent.cgi?referer=https://www.google.com.br/&httpsredir=1&article=1183&context=rhumj
DELAHAYE. Jean-Paul. (2001). Les nombres infinit vers la gauche. Pour la Science, nº 279, 100 – 104. Disponível em: http://www.lifl.fr/~jdelahay/pls/081.pdf
EHRLICH. Philip. (2006). The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Emergence of non-Archimedean Systems of Magnitudes. The Rise of non-Archimedean Mathematics and the Roots of a Misconception I: The Archive History in Exact Sciences. V. 60, 1 – 121. Disponível em: http://prima.lnu.edu.ua/faculty/mechmat/Departments/MFAUKR/attachments/erlich.pdf
FERREIRA, Jamil. (2010). A construção dos números. Textos Universitários. Rio de Janeiro: SBM.
GOLDBLATT, Robert. (1998). Lectures on the Hyprreeals numbers: an introduction to non standard analisys. New York: Springer.
GOUVÊA, F. Q. (1997). p-adic numbers. New York: Springer.
GUSMÃO, Italo, B. (2016). Números p-ádicos. Dissertação de Mestrado Profissional PROFMAT, João Pessoa: UFPB.. Disponível em: https://repositorio.ufpb.br/jspui/bitstream/tede/9337/2/arquivototal.pdf
GAUTSCHI, Walter. Alessandro M. (2002). Ostrowski (1893–1986): la sua vita e le opere. Boll. Docenti Matem. v. 45, 9–19. Disponível em: https://www.cs.purdue.edu/homes/wxg/AMOital.pdf
HASSE, Helmut. (1980). Number Theory. Springer: New York.
HEFEZ, Abramo. (2013). Um curso de Àlgebra, v. 1, Rio de Janeiro: SBM.
HOLLY, Jan E. (2001). Pictures of Ultrametric Spaces, the p-adic Numbers, and Valued Fields. The mathematical association of America. October, 721 – 728. Disponível em: https://www.colby.edu/math/faculty/Faculty_files/hollydir/Holly01.pdf
HUAMAN, Ronald M. (2015). Raıces p-adicas de la unidad. (Tesis de Maestrıa). Pontificia Universidad del Perú: Lima. Disponível em: http://tesis.pucp.edu.pe/repositorio/bitstream/handle/123456789/6415/MAS_HUAMAN_RONALD_PADICAS.pdf;sequence=1
LAGES, Elon. (2010). Curso de Análise, v. 1. Rio de Janeiro: SBM.
LAPIDUS, Michel L.; HUNG. Lu. (2011). The Geometry of p-Adic Fractal Strings: A Comparative Survey. Contemporary Mathematics, v. 551, 163 – 206. Disponível em: http://www.math.ucr.edu/~lapidus/papers/ContMath/GeometrypAdicStringsSurvey10893.pdf
LAPIDUS, Michel L.; HUNG. Lu. (2008). Nonarchimedean Cantor set and string. Journal of Fixed Point Theory and Applications. v. 4, p. 1 – 10. Disponível em: https://pdfs.semanticscholar.org/6dee/45208234f48fe156d174d4ad56b17363d2ef.pdf
LEGUAY, Mathieu; HENRI, Joseph. (1992). Distance p-adique : une distance qui n'est pas habituelle. MATh.en.JEANS” au Palais de la Découverte. 33 – 36. Disponível em: http://mathenjeans.free.fr/amej/edition/actes/actespdf/92033036.pdf
MARCOS, José. E. (2006). The algebraic closure of a p-adic number field is a complete topological field. Mathematica Slovaca,v. 56, No. 3, p. 317—331. Disponível em: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.1010.7259&rep=rep1&type=pdf
MASIAS, Henry Zorrilla. (2011). Complecion no arquimedeana. Pro Mathematica, v. 25, 49-50. Disponível em: http://revistas.pucp.edu.pe/index.php/promathematica/article/viewFile/2666/2610
NARICI, Lawrence; BECKENSTEIN, Edward. (1981). Strange Terrain--Nonarchimedean Spaces. The American Mathematical Monthly, Vol. 88, No. 9 (Nov., 1981), pp. 667-676. Disponível em: https://www.jstor.org/stable/pdf/2320670.pdf
OSTROWSKI, A. (1916). Uber einige L¨osungen der Funktionalgleichung ¨ φ(x)φ(y) = φ(xy)”, Acta Mathematica (2nd ed.), nº 41, no. 1, 271–284. Disponível em: https://projecteuclid.org/euclid.acta/1485887472
KATO, Kazuya; KUROKAWA, Nobushige; SAITO, Takeshi, (2000). Number theory 1: Fermat’s dream, Translations of Mathematical Monographs, vol. 186, American Mathematical Society, Providence, RI.
KATOK, S. (2007). p-adic analysis compared with real. Student mathematical library, American Mathematical Soc.
KOBLITZ, Neal. (1984). p-adic Numbers, p-adic Analysis, and Zeta- Functions. New York: Springer.
KHRENNIKOV, Andrei; KOTOVICHM, Nikolay. (2017). Image Segmentation with the Aid of the p-Adic Metrics. In: TONI, Bourama. New Trends and Advanced Methods in Interdisciplinary Mathematical Sciences. 143 – 155.
KUMAR, Ashish; RANI, Mamta; CHUGH, Renu. (2013). New 5-adic Cantor sets and fractal string. SpringerPlus, 1 – 7. Disponível em: https://link.springer.com/content/pdf/10.1186%2F2193-1801-2-654.pdf
LEWIS, Robert Y. (2019). A formal proof of Hensel’s lemma over the p-adic integers. CPP’19, January p. 14–15, Lisbon, PT. Disponível em: http://robertylewis.com/padics/padics.pdf
NATARAJAN, P N; RANGANATHAN, K. N. (2000). A Geometry in which all Triangles are Isosceles An Introduction to Non-Archimedean Analysis. Resonance, October, 32 – 42. Disponível em: https://www.ias.ac.in/article/fulltext/reso/005/10/0032-0042
NEUKIRCH, J. (1990). The p-Adic numbers. EBBINGHAUS, H.-D. Numbers. New York: Springer. 155 – 179.
OLIVEIRA, Graciano. (2009). O corpo dos p-ádicos. Gazeta Matemática, Dezembro, 7 – 18. Disponível em: http://gazeta.spm.pt/getArtigo?gid=258
ORBEGOSO, Jorge Luis Rojas. Completitud y clausura algebraica de campos P-ádicos. Universidad nacional mayor de san marcos. Perú: Lima, 2016. Disponível em: http://cybertesis.unmsm.edu.pe/bitstream/handle/cybertesis/6315/Rojas_oj.pdf?sequence=1
PITANEN, Matti. (2015). How Imagination Could Be Realized p-Adically?. Journal of Consciousness Exploration & Research, v 6, nº 6, 354 – 356. Disponível em: https://jcer.com/index.php/jcj/article/viewFile/468/488
REDDY, B. Surender; SHANKARAIAH, D. (2013). On i-cauchy sequences in p-adic linear 2-normed spaces. International Journal of Pure and Applied Mathematics. v. 89, nº 4, 483 – 496. Disponivel em: https://ijpam.eu/contents/2013-89-4/4/4.pdf
RIBENBOIM, Paulo. (1999). The Theory of Classical Valuations. New York: Springer.
ROBERT, Alain. (1996). Qu´est-qe que le nombres p- ádique? Societé des enseignants neuchâtelois des Sciences. Bulletin, setembre, 5 – 12. Disponível em: http://www.sens-neuchatel.ch/bulletin/anciens-no-pdf/BULL18.PDF
ROZIKOV, U. A. (2013). What are p-Adic Numbers? What are They Used for?. Asia Pacific Mathematics Newsletter. v. 3, nº 4, 1 – 6, October. Disponível em: http://www.asiapacific-mathnews.com/03/0304/0001_0006.pdf
SCHIKHOF W. H. (1984). Ultrametric Calculus: An introduction to p-adic Analysis. Cambridge: University Press.
SCHLICHENMAIER, Martin. (2007). An Introduction to Riemann Surfaces, Algebraic Curves and Moduli Spaces. Springer: New York.
SILVERMANM, Joseph H. (2013). What is the p-adic Mandelbrot Set?. Notices of the AMS, setember, v. 60, nº 8, 1048 – 1050. Disponível em: http://www.ams.org/notices/201308/201308-full-issue.pdf
STEUDING, Jorn. (2002). The world of p-adic numbers and p-adic functions. Faculty of Physics and Mathematics. nº 5, 90 – 107. Disponível em: http://siauliaims.su.lt/pdfai/2002/STEUD-02.pdf
TEITELBAUM. Jeremy. (1995). The Geometry of p-adic Symmetric Spaces. Notice in American Mathematical Monthly. v. 42, nº 10, 1120 – 1126. Disponível em: https://www.ams.org/notices/199510/teitelbaum.pdf
TELLER, Jacek. (2012). Newton polygons on p-adic number fields. (dissertation in Arts and Mathematics). East Caroline University. Disponível em: http://thescholarship.ecu.edu/bitstream/handle/10342/3848/Teller_ecu_0600M_10676.pdf?sequence=1
TORRES, Sergio Carrillo; AMAYA, Carlos Hurtado. (2001). Una introduccion a los numeros p-adicos. Memorias XVIII encuentro de geometrıa y VI de aritmetica, 359 – 369. Disponível em: http://funes.uniandes.edu.co/5615/1/CarrilloUnaintroduccionGeometr%C3%ADa2008.PDF
VACCON. Tristan. (2015). Précision p-adique. (thésis de doctorat). Rennes: Université de Rennes. Disponível em: https://tel.archives-ouvertes.fr/tel-01205269v2/document
Downloads
Publicado
13-09-2024
Métricas
Visualizações do artigo: 100 PDF downloads: 45
Como Citar
ALVES, Francisco Regis Vieira; OLIVEIRA, Paulo César Cavalcante de. Sobre os números P-ádicos: aspectos históricos, matemáticos e epistemológicos. Revista Brasileira de História da Matemática, São Paulo, v. 24, n. 48, p. 1–32, 2024. DOI: 10.47976/RBHM2024v24n481-32. Disponível em: https://rbhm.org.br/index.php/RBHM/article/view/389. Acesso em: 26 set. 2024.
Edição
Seção
Artigos
