The Alpha Group Tensorial Metric
DOI:
10.47976/RBHM2024v24n4851-57Palavras-chave:
Abstract Algebras, Group Theory, Abstract GeometryResumo
The Alpha Group is an abstract geometry group in R4. The way it was conceived allows a new interpretation of the structure of hypercomplex space with a new geometry and spatial topology, and a meaning for the geometric representation of R4 space to infinity. Therefore, it has been described as the tensorial metric formula in the Alpha Group. It will be shown that the Riemannian and Euclidean distance metrics between infinitesimal surfaces are represented as special cases of the metric of the Alpha group.
Downloads
Métricas
Referências
ANDRONOV, A. A.. Mathematical Problems of the Theory of Self-oscillations. Proc. Vsesojuznaja Konferenziya po Kolebanijam. Moscow. Leningrad. GTTI. 1933.
ARNOLD, V. I.. Teoria da Catástrofe. tradução do Russo: Luiz Alberto P. N. Franco. Editora da UNICAMP, Campinas. 1989.
BOYER, CARL B., História da Matemática. São Paulo: Edgar Blucher, 1996.
CANTOR, G.. Contributions to the Founding of the Theory of Transfinite Numbers (No. 1). Open Court Publishing Company, 1915.
CANTOR, G. Über unendliche, lineare Punktmannigfaltigkeiten, Arbeiten zur Mengenlehre aus dem Jahren 1872–1884. Leipzig, Germany: Teubner, 1884.
COHN, P. M. (1982) Algebra Vol. 1 Second Edition. Bedford College University of London. CORREA, C. S.; de MELO, T. B. e CUSTODIO, D. M.. Proposing the Alpha Group. International Journal for Research in Engineering Application & Management (IJREAM). Volume 08, Issue 05. 2022. doi: 10.35291/2454-9150.2022.0421
HALSTED, G. B. Lobachevsky. The American Mathematical Monthly, v. 2, n. 5, p. 137–139, 1895.
JACOBSON, Nathan (2009), Basic algebra 2 (2nd ed.), Dover, ISBN 978-0-486-47187-7.
KLEIN, F. (1888). Lectures on the Ikosahedron and the Solution of Equations of the Fifth Degree. Trübner & Company.
KLEIN, F. (2004). Elementary mathematics from an advanced standpoint: Arithmetic, algebra, analysis (Vol. 1). Courier Corporation.
POINCARE, H. PHD Thesis, Gauthier - Villars, Paris, 1879.
THOM, R. (1972). Stabilité structurelle et morphogénèse–Essai d’une théorie générale des modèles. Reading Mass.
RIEMANN, G. F. B.; WEYL, Hermann. Über die Hypothesen, welche der Geometrie zu Grunde liegen. Julius Springer, 1923.
WHITNEY, H. (1955). On Singularities of Mappings of Euclidean Spaces. I. Mappings of the Plane into the Plane. Annals of Mathematics, 62(3), 374–410. doi: 10.2307/1970070.
Downloads
Publicado
Métricas
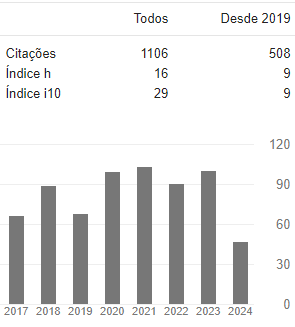
Visualizações do artigo: 272 PDF downloads: 179