Os tratados de George Salmon no contexto da matemática britânica no século XIX: De uma abordagem sintética para uma abordagem analítica
DOI:
10.47976/RBHM2019v19n3845-81Keywords:
Matemática Britânica, George Salmon, Geometria Projetiva, Métodos Projetivos, Coordenadas HomogêneasAbstract
O século XIX é um período divisor de águas na história da humanidade por apresentar os efeitos que a Revolução Industrial provocou na sociedade em geral, onde a Inglaterra foi um dos locais propulsores daquela época. Imersa nesse cenário de transformações, a matemática britânica deste período não ficou atrás em passar também por um processo de mudança. Neste sentido, destaca-se que a abordagem que se fazia presente naquele momento era herdada da tradição de Newton, Taylor e MacLaurin, a qual era fundamentada pela geometria pura ou também conhecida como geometrica sintética. Consciente de que a matemática britânica estava atrasada em relação à matemática dos demais países da Europa, um grupo de matemáticos britânicos formou a Analytical Society para introduzir uma nova abordagem fundamentada na geometria analítica e no cálculo diferencial leibniziano, desenvolvido no século anterior por matemáticos do continente, como Euler, d’Alembert e Lagrange. Esta nova perspectiva, então, proporcionou um avanço na matemática britânica. Essa transição de tratamento se manifesta nos artigos e livros de Peacock, Boole, Sylvester e Cayley, entre outros. George Salmon foi um dos matemáticos a expor essa nova abordagem em tratados didáticos. Se no primeiro tratado de Salmon, percebe-se um viés fortemente sintético, enquanto nos outros tratados posteriores, já se percebe uma abordagem analítica. Destacam-se nos tratados de Salmon os novos resultados dos geômetras do continente, além dos resultados da geometria projetiva que estavam esquecidos por mais de um século.
Downloads
Metrics
References
BALL, Walter William Rouse. 1889. A history of the study of mathematics at Cambridge. Cambridge, Cambridge University Press.
CAYLEY, Arthur. 1845a. Mémoire sur les courbes à double courbure et sur les surfaces développables. In Journal de mathématiques pures et appliquées, 1re série, tome 10, pp. 245-250.
CAYLEY, Arthur. 1845b. Démonstration d’un theorem de M.Chasles. In Journal de mathématiques pures et appliquées, 1re série, tome 10, pp. 383-384.
CAYLEY, Arthur. 1848. On geometrical reciprocity. In Cambridge and Dublin mathematical journal, vol. 3, pp. 173-79, reed. CAYLEY, A. The collected mathematical papers of Arthur Cayley. Cambridge, University Press, vol. 1, 1889. pp. 377-382.
CAYLEY, Arthur.1858b. A fifth Memoir upon Quantics. In Philosophical Transactions of Royal Society of London, reed. CAYLEY, A. The collected mathematical papers of Arthur Cayley. Cambridge, University Press, vol. 2, 1889. pp. 527-557.
CAYLEY, Arthur. 1859. A sixth Memoir upon Quantics. In Philosophical Transactions of Royal Society of London, reed. CAYLEY, A. The collected mathematical papers of Arthur Cayley. Cambridge: At The University Press, vol. 2, 1889. pp. 561-592.
CHASLES. Michel. 1837. Aperçu historique sur l'origine et le développement des méthodes en géométrie. Bruxelles, M. Hayez.
CHASLES. Michel. 1852. Traité de géometrie supérieure, par M. Chasles. Paris, Bachelier, Imprimeur-libraire de l’école polytechine.
CRILLY, Tony. 1986. The Rise of Cayley’s invariant Theory (1841 – 1862). In Historia Mathematica, vol. 13, pp. 241-254.
DIAS, Leandro Silva e GRIMBERG, Gerard Emile. 2015. Álgebra e geometria projetiva analítica na Inglaterra dos anos 1841 – 1853. In Llull, Madrid, v. 38, pp. 11-31.
FISCH, Menachem. 1994. ‘The emergency which has arrived’: the problematic History of Nineteenth-Century British Algebra. In The British Journal for the History of Science, vol. 27, nº. 3, pp. 247-246.
GOW, Rod. 1997. George Salmon 1819 – 1904: his mathematical work and influence. In Irish Math. Soc. Bull. nº. 39. pp. 26-76.
GUICCIARDINI, Niccolò. 1989. The Development of Newtonian Calculus in Britain 1700 – 1800. Cambridge: Cambridge University Press.
JOLY, Charles Jasper. 1905. George Salmon 1819 – 1904. Proc. Royal Society 75, pp. 347-355.
LORENAT, Jemma. 2015. Figures real, imagined, and missing in Poncelet, Plücker, and Gergonne. In Historia Mathematica, Elsevier, pp. 155-192.
LORENAT, Jemma. 2016. Synthetic and analytic geometries in the publications of Jakob Steiner and Julius Plücker (1827–1829). In Archive for History of Exact Sciences, vol. 70, pp. 413-462.
MCCONNELL, Albert Joseph. 1970-1990. Biography of Salmon, in Dictionary of Scientific Biography. New York, Charles Scribner's Sons, vol.12.
MANSION, Paul. 1873. Notice sur les travaux de Jules Plücker. In Bulletin des sciences mathématiques et astronomiques, vol. 5, pp. 313-319.
MENGHINI, Marta. 2019. Luigi Cremona and Wilhelm Fieldler: the link between descriptive and projective geometry in technical instruction. In: Evelyne Barbin, Marta Menghini & Klaus Volkert (org.), Descriptive Geometry - The Spread of a Polytechic Art and the Legacy of Gaspard Monge. Cham: Springer.
MOLLAN, Charles. 2007. It's Part of What We Are: some irish contributors to the development of the chemical and physical sciences (science and irish culture). vol. 2, Hardcover.
PARSHALL, Karen Hunger. 1989. Toward a history of nineteenth-century invariant theory. In: ROWE, D. E.; MCCLEARY, J. The history of modern mathematics. California, San Diego: Academic Press, vol. 1, pp. 157-206.
RICHARDS. Joan. 1986. Projective geometry and mathematica progress in mid-victorian britain. Stud. Hist. Phil. Sci., vol. 17, No. 3, pp. 297–325.
RICHARDS. Joan. 1992. God, Truth, and Mathematics in nineteen century England. The Invention of Physical Science Kluwer Academic Publishers, pp. 51-78.
ROSENFELD, Boris Abramovich. 1988. A History of Non-euclidean Geometry, Evolution of the Concept of a Geometric Space. New York: Springer-Verlag.
SALMON, George. 1846. On the degree of a surface reciprocal to a give one. In Cambridge and Dublin mathematical journal, vol. 2, pp. 65-75.
SALMON, George. 1848. On the condition that a plane should touch a surface along a curve line. In Cambridge and Dublin mathematical journal, vol. 3, pp. 44-46.
SALMON, George. 1848. On the numbers of normals which can be drawn from a given point to a given surface. In Cambridge and Dublin mathematical journal, vol. 3, 1848, pp. 46-47.
SALMON, George. 1852. Théorèmes sur les courbes de troisième degré. In J. für die reine und angewandte Math., pp. 274-276.
SALMON, George. 1850. A treatise on conic sections. 2ª ed, London: Longman, Brown, Green, and Longmans. 3ª ed. London: Longman, Brown, Green, and Longmans, 1855.
SALMON, George. 1851. Sur la formation de l’équation de la courbe reciproque à une courbe donnée. In Journal für die reine und angewandte Mathematik, vol. 42, pp. 277-278.
SALMON, George. 1852. A treatise on the higher plane curves. 1ª ed., Longman, Brown, Green, and Longmans, London. 3ª ed. London: Longman, Brown, Green, and Longmans, 1879.
SALMON, George. 1859. Lessons introductory to the modern higher algebra, Hodge, Smith and Co, Dublin.
SALMON, George. 1862. A treatise on the analytic geometry of three dimensions, Hodge, Smith and Co, Dublin.
SYLVESTER, James Joseph. 1850. On the intersections, contacts, and other correlations of two conics expressed by indeterminate coordinates. In Cambridge and Dublin mathematical journal, vol. 5, pp. 262-285, reed. SYLVESTER, J. J. The collected mathematical papers of James Joseph Sylvester. Cambridge: At The University Press, Vol. 1, 1904. pp. 119-137.
SYLVESTER, James Joseph. 1851. On certain general properties of homogeneous functions. In Cambridge and Dublin mathematical journal, vol. 6, pp. 1-31, reed.
SYLVESTER, J. J. The collected mathematical papers of James Joseph Sylvester. Cambridge: At The University Press, vol. 1, 1904. pp. 165-180.
SYLVESTER, James Joseph. 1851. On the general theory of associated algebraical forms. In Cambridge and Dublin mathematical journal, vol. 6, pp. 289-293, reed.
SYLVESTER, J. J. The collected mathematical papers of James Joseph Sylvester. Cambridge: At The University Press, vol. 1, 1904. pp. 198-202.
VOLKERT, Klaus. 2019. Otto Wilhelm Fiedler and the synthesis of projective and descriptive geometry. In: Evelyne Barbin, Marta Menghini & Klaus Volkert (org.), Descriptive Geometry - The Spread of a Polytechic Art and the Legacy of Gaspard Monge. Cham: Springer, pp. 168-181.
WILSON, Edwin Bidwell. 1903. The synthetic treatment of conics at ter present time. In Bull. Amer. Math. Soc.vol. 9, nº 5, pp. 248-254.
Downloads
Published
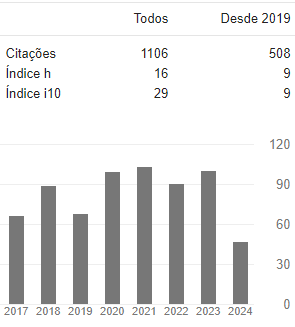
Métricas
Visualizações do artigo: 546 PDF (Português (Brasil)) downloads: 359