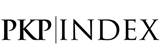A Sequência de Padovan ou de Coordonier
DOI:
10.47976/RBHM2022v22n4521-43Keywords:
Números de Padovan, Sequência, Evolução histórica, Definições matemáticasAbstract
De modo geral, os autores de livros de História da Matemática dedicam um expediente de discussão considerável aos números de Fibonacci. De fato, uma profusão de exemplos e sua manifestação, por intermédio de propriedades, de toda ordem, parecem eclipsar o papel e a constatação da ocorrência pitoresca de outras entidades matemáticas numéricas. Por conseguinte, o presente trabalho busca envidar uma discussão em torno do número plástico e da sequência de Padovan. Seu espaço de utilidade singular foi detectado na Arquitetura, todavia, por intermédio de um processo matemático evolutivo, histórico e epistemológico, o trabalho constata o interesse atual na pesquisa em Matemática, cujo vigor evolutivo pode ser objetivado por intermédio do estabelecimento de novas definições matemáticas que representam, grosso modo, seu processo ininterrupto de formalização e de generalização. Isso posto, propugnam-se, ao final do mesmo, importantes e significativas repercussões e uma legítima trajetória epistemológica evolutiva que comunica ao leitor um viés não estático e evolutivo do pensamento e dos objetos matemáticos.
Downloads
Metrics
References
ALSINA, Claudi.; NELSEN, Roger. B. A mathematical space odyssey: solid geometry in the 21th Century. The mathematical association of America. 2015.
ALSINA, C. J.L. GARCÍA-ROIG: On plastic numbers. Journ. Math.& Design, Vol.1 No.1 (2001), p.13-19. Disponível em: http://www.ajmao.org/WebEditor/Pagines/el%20numero%20de%20oro%20es%20plano.pdf
ALVES, Francisco. R. V. Fórmula de de moivre, ou de binet ou de lamé: demonstrações e generalidades sobre a sequência generalizada de fibonacci – SGF. Revista Brasileira de História da Matemática, v. 17, nº 33, 1 – 16. 2017. Disponível em: http://www.rbhm.org.br/issues/RBHM%20-%20vol.17,no33/Francisco.pdf
AARTS, Jan; FOKKINK, Robbert; KRUIJTZER, Godfried. Morphic numbers. Nieuw Archief voor Wiskunde, v. 5, nº2, p. 56–58, 2001. Disponível em: http://www.nieuwarchief.nl/serie5/pdf/naw5-2001-02-1-056.pdf
AUDET. Daniel. Le nombre plastique: cousin negligé du nonbre d´or. Association mathematique du Quebec. v. 53, nº 1, 29 36. 2013. mars, Disponível em: http://archimede.mat.ulaval.ca/amq/bulletins/mar13/9-maitre-Plastique-mars-2013.pdf
BUGATTI, Enrica Caterina. Dom Hans van der Laan e il numero plastico. Corso di Laurea in Architettura. Milano. 2012. Disponível em: https://www.politesi.polimi.it/bitstream/10589/57542/1/2012_07_Bugatti.pdf
BUITRAGO, A. Redondo. Los numeros morficos en secundaria. REVISTA SUMA. 59, 7–16, 2008. Disponível em: http://www.ajmao.org/WebEditor/Pagines/los%20numeros%20morficos%20en%20secundaria.pdf
CHOULET. Richard. Après le nombre d’or, quels nombres aussi riches inventer ? Revue Mathématique et Pédagogie. nº 166, 3 – 30. 2008. Disponível em: https://www.apmep.fr/IMG/pdf/nbag.pdf
GAZALÉ, Midhat J. GNOMON - From Pharaohs to Fractals. Princenton: Princeton University Press. 1999.
GUTIÉRREZ, V. Sainz. La arquitectura monástica de Dom Hans van der Laan. Actas del Congreso Internacional de Arquitectura Religiosa Contemporánea. 86 – 107, 2009. Disponível em: https://idus.us.es/xmlui/handle/11441/25348
IIIOUPOULOS, Vasileios. The plastic number and its generalised polynomial. AirxiV. 1 – 4, 2014. Disponível em: https://www.researchgate.net/publication/263738896_The_plastic_number_and_its_generalized_polynomial
KOVACIC, Bojan; MAROHNIC, Luka; OPACIC, Renata. (2013). O Padovanovu nizu. Osjecki Matematicki. Croacia, v. 13, nº 1. Available in: https://bib.irb.hr/prikazi-rad?rad=628833
MAROHNIĆ, Luka; KOVAČIĆ, Bojan; RADIŠIĆ, Bojan. O nultočkama polinoma oblika . Croacia, Osječki matematički list. nº 13, 1 – 13. 2013. Disponível em: http://bib.irb.hr/datoteka/749107.O_nultokama_polinoma_xn-x-1.pdf
MAROHNIĆ, Luka; STRMEČKI, Tihana. Plastic Number: Construction and Applications. Advanced Research in Scientific Areas. December, 3 – 7, 2012. Disponível em: https://bib.irb.hr/datoteka/628836.Plastic_Number_-_Construct.pdf
GONZÁLEZ-DÍAZ, María-Jesús; GARCÍA-NAVARRO, Justo. Non-technical approach to the challenges of ecological architecture: Learning from Van der Laan. Frontiers of Architectural Research, v. 5, 202 – 212. 2016. Disponível em: https://ac.els-cdn.com/S2095263516000121/1-s2.0-S2095263516000121-main.pdf?_tid=018e396c-06db-4d3d-8485-03824cdf0f40&acdnat=1526491904_907780ce59b4bf45cc3fca0b6773df3b
JAVIER, Rodrigo; DOLOREZ, L. Generalizaciones de los números de oro y Padovan. Segundo Congreso Internacional de Matemáticas en la Ingeniería y la Arquitectura. 407 – 422, Madrid. 2008. Disponível em: http://www2.caminos.upm.es/Departamentos/matematicas/Fdistancia/MAIC/CONGRESOS/SEGUNDO/028%20Generalizaciones.pdf
PADOVAN. Richard. Dom Hans Van Der Laan and the Plastic Number, p. 181-193. Nexus IV: Architecture and Mathematics, eds. Kim Williams and Jose Francisco Rodrigues Fucecchio (Florence): Kim Williams Books, 2002. Disponível em: http://www.nexusjournal.com/the-nexus-conferences/nexus-2002/148-n2002-padovan.html
PIMM, David.; SINCLAIR, Nathalie. Aesthetics and Mathematical Mind. In: PIMM, David.; SINCLAIR, Nathalie; HIGGINSON, William. Mathematics and Aesthetics: new approaches to the Ancient Infinity. New York: Springeg. p. 223 – 255. 2000.
SELMER, E. S., On the irreducibility of certain trinomials. Mathematica. Scand. v. 4, 287–302. 1956. Disponível em: http://www.mscand.dk/article/view/10478/8499
SAHIN, Adem. On the generalized perrin and cordonnier. Commun. Fac. Sci. Univ. Ank. SÈrvia. v. 68, nº 1, 242 – 253. 2017. Disponível em: http://dergiler.ankara.edu.tr/dergiler/29/2136/22111.pdf
SOKHUMA, Kritsana. Matrices formula for Padovan and Perrin Sequences. Applied Mathematical Sciences. v. 7, nº 142, 7093 – 7096, 2013. Disponível em: https://pdfs.semanticscholar.org/99b4/476c10c2e36f1292e8333d088b33dcdba0ea.pdf
SOUSA GOMES, Vasco. M. V. Sistemas proporcionais como metodologia de sistematização projecutal. (Dissertação de mestrado em Arquitetura). Porto: Faculdade de Arquitetura do Porto. 2012. Disponível em: https://repositorio-aberto.up.pt/bitstream/10216/80297/2/23453.pdf
SPINADEL, Vera W.; BUITRAGO, Antonia Redondo. Towards van der Laan’s Plastic Number in the Plane. Journal for Geometry and Graphics. v. 13, nº 2, 163 – 175, 2009. Disponível em: http://www.heldermann-verlag.de/jgg/jgg13/j13h2spin.pdf
SPINADEL, Vera. W. La familia de numeros metalicos. Cuadernos del CIMBAGE, nº 6, 17 – 44, 1999. Disponível em: http://www.redalyc.org/html/462/46200602/
STEWART, Ian. Professor Stewart’s Cabinet of Mathematical Curiosities. New York: Basic Books. 2008.
STEWART, Ian. L´Univers des Nombres. Paris: Belin pour la Science. 2000.
TASCI. Dursun. Gaussian padovan and gaussian pell- padovan sequences. Commun. Fac. Sci. Univ. Ank. Ser. A1 Math. Stat. v. 67, nº 2, 82 – 88. 2018a. Disponível em: http://dergiler.ankara.edu.tr/dergiler/29/2239/23280.pdf
TASCI. Dursun. Padovan and pell-padovan quaternions. Journal of Science and Arts. v. 18, nº 1, 125 – 132. 2018b. Disponível em: http://www.icstm.ro/DOCS/josa/josa_2018_1/a_08_Tasci_125-132.pdf
VOET, Caroline; SCHOONJANS, Yves. Benidictine thought as a catalist for 20™ century liturgical space: the motivation behind Dom Hans Van der Laan´s aesthetic church arquitectury. Proceeding of the 2nd international conference of the Europa Architetural History of Network. May, 255 – 261, 2012. Disponível em: https://lirias.kuleuven.be/bitstream/123456789/391810/1/C+VOET+eahn+2012.pdf
YILMAZ, Nazmiye. Padovan ve perrin sayilarinin matris temsilleri. (Doctorat thesis). Konya: Turky University. 2015. Disponível em: http://acikerisim.selcuk.edu.tr:8080/xmlui/bitstream/handle/123456789/3682/418927.pdf?sequence=1&isAllowed=y
YILMAZ, Nazmiye; TASKARA, Necati. Matrix Sequences in terms of Padovan and Perrin Numbers. Journal of Applied Mathematics. v. 7, 1 – 7. 2013. Disponível em: https://www.hindawi.com/journals/jam/2013/941673/
YILMAZ, Nazmiye; TASKARA, Necati. On the Negatively Subscripted Padovan and Perrin Matrix Sequences. v. 5, nº 2, 59 – 72. 2014. Disponível em: http://www.rgnpublications.com/journals/index.php/cma/article/view/227
WEISS, Gunter. From the golden rectangle to laan’s box and more. Proceedings of 21st conference geometry graphics computer. p. 81 – 82, 2014a. Disponível em: http://ogigi.polsl.pl/zeszyt_Gdansk_14/81_proceeding_2014.pdf
WEISS, Gunter. Solving algebraic equations with Fibonacci sequences. Proceedings of the 9th International Conference on Applied Informatics. Hungary, February, v. 1, 9 – 18. 2014b. Disponível em: http://icai.ektf.hu/icai2014/papers/ICAI.9.2014.1.9.pdf
WEGER, Benjamin M. M. Padua and pisa are exponentially far apart. Publications Matemàtique. v. 41, 631 – 651, 1997. Disponível em: http://www.jstor.org/stable/pdf/43737267.pdf?refreqid=excelsior%3A6a7108e9bb19ca16ee6473d7d16e9754
Downloads
Published
Métricas
Visualizações do artigo: 1119 PDF (Português (Brasil)) downloads: 583