O INFINITO E O MÉTODO DA DIAGONAL DE CANTOR – TRADUÇÃO DE UEBER EINE ELEMENTARE FRAGE DER MANNIGFALTIGKEITSLEHRE (1890–91)
DOI:
10.47976/RBHM2023v23n46421-439Palavras-chave:
Infinito, G. Cantor, Método da Diagonal, TraduçãoResumo
No presente artigo, apresentamos a tradução ao português e a transcrição alemã do artigo intitulado “Ueber eine elementare Frage der Mannigfaltigkeitslehre” (1890–91), no qual Cantor introduz o seu famoso “Método da Diagonal”. No ensaio introdutório, fazemos algumas considerações acerca do infinito e dos problemas correlatos, bem como apresentamos alguns elementos para colocar a história do infinito numa perspectiva cultural mais ampla.
Downloads
Métricas
Referências
BERTATO, F. M. Bolzano on God as the ground of the existence of the actual infinite. 2023. No prelo.
BERTATO, F. M. Philosophical Approaches to the Foundations of Logic and Mathematics: In Honor of Professor Stanisław Krajewski. Review. History and Philosophy of Logic, págs. 1–3, 2022. <https://doi.org/10.1080/01445340.2022.2083906>.
BOLZANO, B. Lehrbuch der Religionswissenschaft. 3 Vol. Sulzbach: Seidel, 1834.
BOLZANO, B. Paradoxien des Unendlichen. Leipzig: C. H. Reclam Sen, 1851.
BOLZANO, B. Paradoxes of the Infinite. Transl. Donald A. Steele. London: Routledge and Kegan Paul, 1950.
BOLZANO, B. Las Paradojas del Infinito. Transl. Luis Felipe Segura. Mexico: Mathema, 2005.
BRITO, A.; BERTATO, F. M. Infinito e enumerabilidade: uma apresentação do trabalho inaugural de Cantor. Revista Brasileira de História da Matemática, 2023. No Prelo.
CANTOR, G. Ueber eine Eigenschaft des Inbegriffes aller reellen algebraischen Zahlen. Journal für die reine und angewandte Mathematik. Vol. 77, págs. 258–262. 1874.
CANTOR, G. Ueber eine elementare Frage der Mannigfaltigkeitslehre. 1890–91. In: ZERMELO, E. Georg Cantor: Gesammelte Abhandlungen mathematischen und philosophischen Inhalts. Hildesheim: Olms, págs. 278–281, 1962.
CANTOR, G. Ueber eine elementare Frage der Mannigfaltigkeitslehre. Jahresbericht der Deutsch. Math. Vereing. Vol. I, págs. 75–78, 1890–91.
CORTESE, J. Infini et disproportion chez Pascal. Paris: Honoré Champion, 2023
DAUBEN, J. W. Georg Cantor: His Mathematics and Philosophy of the Infinite. Princeton, New Jersey: Princeton University Press, 1990.
DAVENPORT, A. Measure of a different greatness: The intensive infinite, 1250–1560. Leiden: Brill, 1999.
DEDEKIND, R. Was sind und was sollen die Zahlen? Braunschweig: Druck und Verlag von Friedrich Vieweg und Sohn, 1893.
DEDEKIND, R. Essays on the Theory of Numbers: I. Continuity and Irrational Numbers: II. The Nature and Meaning of Numbers. Trad. Wooster Woodruff Beman. Chicago: The Open Court Publ. Co., 1909.
EPSTEIN, R. L.; CARNIELLI, W. A. Computability. Computable Functions, Logic, and the Foundations of Mathematics, 3rd Edition. Socorro, New Mexico: Advanced Reasoning Forum, 2008.
EWALD, W. B. From Kant to Hilbert: A Source Book in the Foundations of Mathematics. Volume 1. Oxford: Oxford University Press, 2005.
FERREIRÓS, J. Labyrinth of Thought. A history of set theory and its role in modern mathematics. Basel, Boston: Birkhäuser Verlag, 2001.
GALILEU GALILEI. Discorsi, e dimostrazioni matematiche, intorno à due nuoue ſcienze, Attenenti alla Mecanica, & i Mouimenti Locali. Bologna: HH de Dozza, 1654.
LINTZ, R. G. Considerações Gerais sobre a Filosofia da História e sua Influência na Evolução das Formas Matemáticas. Bolema, Rio Claro – SP, v. 7, n. ESPECIAL 2, pp. 84–92, 1992.
LINTZ, R. G. História da Matemática. 2 Vols. (45 e 46 da Coleção CLE). Campinas: CLE, 2017.
MURAWSKI, R. On the Reception of Cantor's Theory of Infinity (Mathematicians vs. Theologians). In: Philosophical Approaches to the Foundations of Logic and Mathematics: In Honor of Professor Stanisław Krajewski. Poznań Studiesin the Philosophy of the Sciences and the Humanities, 114. Leiden, The Netherlands,Brill/Rodopi, 2021.
PARIKH, J. Ten Primary Upanishads: Word-for-Word Translation from Sanskrit. [sl]: Notion Press, 2022.
PLATÃO. Diálogos. Vol. I. Madrid: Gredos, 1993.
SHAPIRO, S.; HELLMAN, G. T. The History of Continua: Philosophical and Mathematical Perspectives. Oxford: Oxford University Press, Year: 2021.
SIEG, W. Hilbert’s Programs and Beyond. Oxford: Oxford University Press, 2013.
SUPPES, P. Axiomatic Set Theory. Princeton: D. Vand Nostrand, 1960.
SWEENEY, L. Divine Infinity in Greek and Medieval Thought. New York: Peter Lang, 1992.
VENTURI, G. Cantor E L’infinito. Lett. Mat. Pristem 107, 61–69, 2018. https://doi.org/10.1007/s10031-018-0038-x.
ZELLINI, P. Breve storia dell’infinito. Milano: Adelphi, 1980.
ZERMELO, E. Georg Cantor: Gesammelte Abhandlungen mathematischen und philosophischen Inhalts. Hildesheim: Olms, 1962.
Downloads
Publicado
Métricas
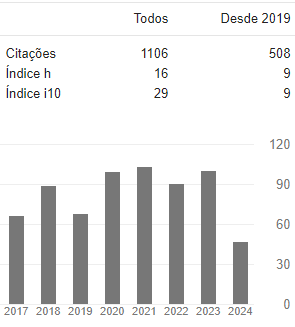
Visualizações do artigo: 452 PDF downloads: 369